바나흐 공간

수학에서, 특히 함수 해석에서, 바나흐 공간([banbanax]로 발음됨)은 완전한 규범 벡터 공간이다.따라서, 바나흐 공간은 벡터 길이와 벡터 사이의 거리를 계산할 수 있는 메트릭을 가진 벡터 공간이며, 코시 시퀀스가 항상 공간 내에서 잘 정의된 한계로 수렴한다는 점에서 완성됩니다.

바나흐 공간은 한스 한, 에두아르트 헬리와 ^[1]함께 1920~1922년 이 개념을 도입하고 체계적으로 연구한 폴란드 수학자 스테판 바나흐의 이름을 따왔다.모리스 르네 프레셰는 "바나흐 공간"이라는 용어를 처음 사용했고 바나흐는 "프레셰 공간"^[2]이라는 용어를 만들었다.바나흐 공간은 원래 21세기 초에 힐베르트, 프레셰, 리즈에 의해 함수 공간에 대한 연구를 통해 성장했습니다.바나흐 공간은 함수 분석에서 중심 역할을 합니다.분석의 다른 영역에서는 연구 중인 공간이 Banach 공간인 경우가 많습니다.

정의.

A바나흐 공간은 완벽한normed 공간(X,‖ ⋅ ‖).normed 공간{\displaystyle(X,\ \cdot)).}은 pair[노트 1](X,‖ ⋅ ‖){\displaystyle(X,\ \cdot))} 스칼라장 K{\displaystyle \mathbb{K}에 대한 벡터 공간으로 구성된 X{X\displaystyle}}(어디 K{\displaystyle \mathbb{K}}. comm은오직 R{\displaystyle \mathbb{R}}또는 C{\displaystyle \mathbb{C}})같이 distinguished[주 2]규범‖⋅ ‖:X→ R.{\displaystyle\와 같이 \cdot\:X\to \mathbb{R}.}모든 표준처럼, 생태계가 번역 invariant[주 3]거리 함수 또는(규범)유도 정준 미터 법이라고 불리는 -LSB- n.에 의해 정의됨이다ote 4]

모든 $벡터{\displaystyle }$ x${\displaystyle ,}$ ${\displaystyle y}$ ${\displaystyle †}$ $.$ {{$displaystyle$ x$,y\in$ X$.}$ 그러면$$ X$${\$displaystyle$ X$}$가 미터법${\displaystyle \mathrm{공간}(X,}$d${\displaystyle )}$으로 $바뀝니다{\displaystyle }$$.\displaystyle (X,d)$}축차)∙)()n)nx1∞{\displaystyle x_{\bullet}(x_{n}\right)_{n=1}^{\infty}}라고 불린다 d{\displaystyle d}또는 코시(X, d){\displaystyle(X,d)}또는 ‖⋅ ‖에{\displaystyle\와 같이 \cdot)}-Cauchy-Cauchy 모든 진짜 r>;0,{\displaystyle r>0,}이 s존재하ome에$덱스{\displaystyle }$ N$(\displaystyle$ N$)$은 다음과 같습니다. m$${\$displaystyle$ m$}$ 및$$$n{\displaystyle }$ {$displaystyle$ n$}$이 $N$보다$$클 때마다 $표준{\displaystyle }$ 메트릭 d$${$displaystyle$$$n$}$ 쌍$,$$)$이 완전한 메트릭 공간인 경우 표준 $메트릭{\displaystyle }$ d {$displaystyle$ d$}$는 $완전$한 메트릭이라고 불립니다.${\displaystyle {}_{}}$ x${\$${\displaystyle {}_{}^{}{}_{}{{}_{}}_{}^{}}$( ${\displaystyle {x_{}}_{}^{}}$ ${\displaystyle {}_{}^{}}$ ) ${\displaystyle {\mathrm{n}}_{}^{}}$ ${\displaystyle {=}_{}^{}}$ 1$$ { $display style$x _ { \ $right$} _ { $n =$ { $n }^{$ \ $infty }$ in$$ (${\displaystyle X}$ , $d$ ) 、 { $display style$( X ,$$d ) ${\displaystyle \}}$${\displaystyle }$、 { $display style$x \ $in$X } } ${\displaystyle \mathrm{some<img\; alt="\{\backslash displaystyle\; (X,d),\}"\; aria-hidden="true"\; class="mwe-math-fallback-image-inline"\; src="https://wikimedia.org/api/rest\_v1/media/math/render/svg/65d3f7e7ed7282e6a27730d9748c58fe2667a87e"\; style="vertical-align:\; -0.838ex;\; width:6.686ex;\; height:2.843ex;">}}$ some$some{\displaystyle }$ x x x x x some some1 。${\displaystyle {여기}_{}}$서${\displaystyle }$x${\displaystyle {}_{}}$n - ${\displaystyle x}$ ${\displaystyle \Vert }$ ${\displaystyle =d}$ ( ${\displaystyle x_{}}$n ,${\displaystyle x}$) ,$${ $displaystyle$ \ left \ $x$_ { $n$} -$x$ \$right$ \ =$$d \$left$( $x$_ {$n$ ,$x$ \ $right )$$$} 이$$ x { $displaystyle$x } 로의 수렴은 다음과 같이 나타낼 수 있습니다.

정의상 $표준유도메트릭{\displaystyle }$ d$\displaystyle$$$d$\$displaystyle$$d\cdot$\)$가${\displaystyle }$완전메트릭인 경우 표준공간$X$d${\displaystyle )}$은 Banach 공간입니다.또, (${\displaystyle X,}$ $)\displaystyle(X, d)$가 완전메트릭공간인 경우에는${\displaystyle }$달리 설명하겠습니다.표준공간$({\displaystyle X,}$ X$,$ \$cdot\)$의 표준공간$(\$style$\$$display\cdot\)$은 (${\displaystyle X,}$ \cdot$\)\$displaystyle$(X,$ \$cdot\)$이 바나치공간일${\displaystyle }$경우 완전표준이라고 불립니다.

L-반내 제품

X$\$$displaystyle$$($$X$\$cdot$ \$$$\$$displaystyle$$\sqrt{(}$X$,\$$cdot)$에 대해${\displaystyle }$X$\$ X에 L-semi-inner 제품${\displaystyle ⟩⟩}$ $、\sqrt{}$$$$⟩$ 、 ⟩⟩⟩⟩⟩ 、 ⟩⟩ ⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩ $\sqrt{⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩⟩}$에랄, 이 조건을 충족하는 L-semi-inner 제품은 무한히 많을 수 있습니다.L-semi-inner 제품은 힐버트 공간을 다른 바나흐 공간과 근본적으로 구별하는 내부 제품의 일반화입니다.이는 모든 표준 공간(따라서 모든 Banach 공간)이 (이전) Hilbert 공간의 일반화라고 간주할 수 있음을 나타냅니다.

시리즈에 따른 특성화

벡터 공간 구조를 사용하면 일련의 벡터를 수렴하는 것과 코시 시퀀스의 동작을 연관시킬 수 있습니다.표준 $공간{\displaystyle }$X({$displaystyle$X})는$$ X$({displaystyle$X})의 각 절대 수렴 시리즈가 X$({displaystyle$ X$})$로 수렴되는$$ 경우에만 Banach 공간입니다.

토폴로지

표준공간$({\displaystyle X,}$ "${\displaystyle }$"${\displaystyle }$" "${\displaystyle }$" )의 $표준메트릭{\displaystyle }$ d$(\$$displaystyle$$$( X$, \cdot$\ )는$$$$ X${\displaystyle ,}$\$displaystyle$ X의 표준메트릭 토폴로지 $(\$ _${d$})를 유도합니다$.$여기서 이 토폴로지는 표준 또는 표준 유도 토폴로지라고 불립니다.특별히 지시되지 않는 한 모든 표준 공간은 자동으로 이 하우스도르프 토폴로지를 전송하는 것으로 간주됩니다.이 토폴로지에서는 모든 ^[4]바나흐 공간은 Baire 공간이지만 Banach가 아닌 표준 공간이 존재합니다.노름 ${\displaystyle \Vert \Vert }$(${\displaystyle }$:${\displaystyle }$( X , ${\displaystyle d_{}d}$ ) $→$ ${\displaystyle R\mathbb{}}$ \ \ $displaystyle \$, \ $cdot$\ , \ : $\$ left ( $X$, \ $tau$_ {$d}$ \ $right$)$$\ \ \ $mathbb {R }$는 유도되는 토폴로지에 관한 연속 함수입니다.

x ${\displaystyle †}$ $(\displaystyle$x\$in$ X$)$를 중심으로 한 r> (\$displaystyle r>$ 0)의 오픈볼과 클로즈볼은 각각 세트입니다.

이러한 공은${\displaystyle }X$의$$볼록하고 $유계된$ 부분 $집합$이지만$,$ X가$$유한 차원 벡터 공간인 $경우$에만 콤팩트한 공/근방이 존재합니다.특히 무한 차원 규범 공간은 국소적으로 콤팩트하거나 하이네-보렐 특성을 가질 수 없다.${\displaystyle {x\mathrm{}}_{}}$ 0$(\$이 $벡터$이고 s${\displaystyle 0}$0(\$displaystyle$ s$\neq$ 0$)$이 스칼라일 $경우{\displaystyle {}_{}}$s : $1$ ( \ $displaystyle$ s : ${\displaystyle =}$ 1 )을 $사용$하면 이 표준유도 토폴로지가 변환불변임을 알 수 있습니다.즉$,$ X$$( \ $displaystyle$x \ $in$X $)$ S${\displaystyle }$( \ $displaystyle$S \ $subseteq$X$$)의 $서브셋{\displaystyle }$S ( \ $displaystyle$$$S )가$$$$ 이 X에서 $열려{\displaystyle }$ 있는 경우에만 $열립니다$.f $변환{\displaystyle }$x +${\displaystyle S}$ : ${\displaystyle =}$ {${\displaystyle x}$ +${\displaystyle s}$ : ${\displaystyle ss}$ S ${\displaystyle \}}$. { $displaystyle$x + S : = \ {$x + s$ :$s$ \ $in$ S \$$} 결과적으로$$ 표준 유도 토폴로지는 원점 근방 기준으로 완전히 결정됩니다.발신기지에는 다음과 같은 일반적인 네이버베이스가 있습니다.${\displaystyle {{}_{}}_{}^{}}$서${\displaystyle {}_{}^{}}$( ${\displaystyle {r_{}}_{}^{}}$n ) ${\displaystyle {}_{}^{}}$ ${\displaystyle {=}_{}^{}}$ ${\displaystyle 1_{}^{}}$ { $displaystyle \left$($r$_ { $n$} \ ${\displaystyle {right}_{}}$ $)_{n=1}^{\infty$}}은$$ R$${\$displaystyle$$$$\mathbbb {R}$ $$$}$의$0$($예{\displaystyle {}_{}}$: ${\displaystyle r_{}}$ :${\displaystyle 1}$/ ${\displaystyle n}$ \ $displaystyle r_n$${\displaystyle =}$$$${\displaystyle 1}$/ n )으로 수렴하는 양의 실수열입니다.$예$를 들어$$ n$}).$예를 들어$$X의$$ $모든{\displaystyle }$ 열린 부분 $집합{\displaystyle }$ U$({$$displaystyle$U$})$는 유니언으로 쓸 수 있습니다. 모든 r){\displaystyle r_{)}}형태 r의 나는 U⊆ 일부 부분 집합에 의해 Indexed{\displaystyle I\subseteq U,})=1nx{\displaystyle r_{)}={\tfrac{1}{n_{)}}}}일부 정수 nx>0{\displaystyle n_{)}>. 0}일 경우(는 닫힌 공 또한 개방된 공이 아니라, 그 비록 사용될 수 있다. 지수ing $세트{\displaystyle }$ I$(Displaystyle$ I) $radii$ r $x($Displaystyle r_${x$})를$$ 변경해야 할 수 있습니다.또한 X$(\$$displaystyle$ X$)$가 분리 가능한 공간인 경우 $언제$든지$$I(\$displaystyle$ I$)$를 계수 가능한 고밀도 서브셋으로$선택$할 수 있습니다.Anderson-Kadec 정리에 따르면, 모든 무한 차원 분리 가능한 프레셰 공간은 $\underset{}{곱}$ 공간$\underset{}{}$θ i$\underset{}{n\mathbb{}}$ N $\underset{}{R}\mathbb{}$$${\$textstyle$$$\$prod$ _${i\in \$$mathbb$${N}}\mathbb {$$R}$ $$$}$에 동형이다(이 ^[5]맵은 선형 동형이 필요 없음).모든 Banach 공간은 Fréchet 공간이기 때문에 이는 분리 가능한 Hilbert ${\$ \$displaystyle \ell$} 시퀀스² 공간 ${\displaystyle {2\mathrm{}}^{}}$2${\displaystyle {}_{}}$( ${\displaystyle N\mathbb{}}$) \ $displaystyle$$\ell$ ^ {$N$} ( \$mathbbb$ {$N$$}$ )를 포함한 $모든$ 무한 차원 분리 가능한 Banach 공간에도 해당됩니다$.$유한 차원 공간에 대한 온트라스트) ${\displaystyle {2\mathrm{}}^{}}$2 ( $N$) 2 2 ( \$displaystyle$ \$ell$^${2$} ( \ $mathbb$ { N$$} )는 ${\displaystyle {단위구\mathrm{}}^{}}${ ${\displaystyle x}$∈${\displaystyle 2\mathbb{}}$ ( N${\displaystyle }$) :${\displaystyle {x\mathrm{}}_{}}$ $2$ ${\displaystyle 1}$} . { $displaystyle$ \$left$\ { $x\in$ \ $^2}$ ( $mathbbb { N$} ) = { 1 } x 2 } \ { 1 } } } } = { 1 } } }에도 동형식입니다.$}$

이 규범에 의해 유도되는 토폴로지는 (${\displaystyle X,}$ ${\displaystyle {\delta }_{}}$ d$)$ \$displaystyle \left(X,\tau$ _${d}\right)$를${\displaystyle }$topology vector space(TVS; 토폴로지 벡터 공간)로 알려져 있습니다.이것은 정의상 덧셈과 스칼라 곱셈의 연산을 연속적으로 하는 토폴로지가 부여된 벡터 공간입니다.TVS $({\displaystyle }$ ${\displaystyle X}$, ${\displaystyle d_{}}$d $){$ $displaystyle \left$($X$, \ $tau$_ {$d$} \ $right$)는$$ 특정 유형의 토폴로지와 함께 벡터 공간일 뿐이라는 점이 강조되고 있습니다.즉, TVS로 간주되는 경우, 이 공간은 특정 표준 또는 메트릭(둘 다 "잊혀짐")과는 관련이 없습니다.

완전한 계량 가능한 벡터 토폴로지의 비교

The open mapping theorem implies that if ${\displaystyle \tau {\text{ and }}\tau _{2}}$ are topologies on ${\displaystyle X}$ that make both ${\displaystyle (X,\tau )}$ and ${\displaystyle \left(X,\tau _{2}\right)}$ into complete metrizable TVS (for example, Banach or Fréchet 공백) 및 한쪽 토폴로지가 다른 쪽 토폴로지보다 가늘거나 거칠면 같아야 합니다(즉, "${\displaystyle \text{2}{}_{}"}$ 또는 "${\displaystyle {2\mathrm{}}_{}}$가 ${\displaystyle 같으면}$ "2$"$ $=$ "$"$ (\$display$\display \$dispeq$ \$display$ \dispect_${\$text${}\dispecteq\dispect_{\text{})$^[6]{\$text${}}} $={\disparam$})예를 들어 (${\displaystyle X}$ ,${\displaystyle p}$ )및 ${\displaystyle (X}$ ,${\displaystyle q}$ $){$ $displaystyle ( X$ , p$){$ \$text$ { $and }}$ ($X$ ,$q$ )}이$$ 토폴로지가 ${\displaystyle p_{}}$ ${\displaystyle \text{및}{}_{}}$ { $displaystyle$\ $tau _$ { $p$}{\$text${\$text$ { $}$ 및$$ }인 경우$,{\displaystyle }$ 그리고 이들 공간 중 하나가 볼의 서브셋이 열려 있는 경우${\displaystyle q_{}}$q )${\displaystyle }$→$R$ {{$display style p$:\$left(X,\$$tau$$_${q}\right${\displaystyle )\backslash }$$to \mathbb$ {R$}}$ ${\displaystyle 또는{}_{}}$ : (X,${\displaystyle {}_{}\tau \mathbb{}}$p )→ R${\displaystyle q:\left(X,\tau$ _{$p}\right)\to$ \mathbbb {R$$$}$ 동일하며 그 토폴로지가 됩니다.

완전성

완전한 규범과 동등한 규범

벡터 공간상의 두 가지 규범은 동일한 ^[7]위상을 유도하는 경우에만 동등하다고 불립니다.$p{\displaystyle }$$\displaystyle$p와 q$$$\$$displaystyle$q가$$ 벡터 $공간{\displaystyle }$X(\$displaystyle$ X)의$$ 두 가지 동등한 규범인 $경우{\displaystyle }$$({\displaystyle X,}$$)\displaystyle(X,$${\displaystyle p}$$)\$$displaystyle(X,q)\$displaystyle(X,q)\displaystyle(\displaystyle)가$$ 바나흐 공간인 경우에만)이$$ 됩니다.바나흐 공간의 연속 노름 예시로 바나흐 공간의 주어진 ^{[note 5][7]}노름과 동일하지 않은 것을 보려면 이 각주를 참조하십시오.유한 차원 벡터 공간의 모든 규범은 동일하며 모든 유한 차원 규범 공간은 바나흐 ^[8]공간이다.

완전한 기준과 완전한 메트릭

A미터 DX{X\displaystyle}X{X\displaystyle}에 기준을 모든 sca에 D(s), sy))이란 말예요. D(), y){\displaystyle D(sx,sy)=의 D(x, y)} 되다면 D{D\displaystyle}은 번역 invariant[주 3]고 완전히 균질에 의해 유도된 벡터 공간에{D\displaystyle}.lars ${\displaystyle s}${\$displaystyle$ s$}$ 및$$$$ $all{\displaystyle }$x , ${\displaystyle y}$ ${\displaystyle \in X}$, { $displaystyle$x ,$y$ \ $in$ X$$} 。이 경우${\displaystyle }$함수 "${\displaystyle x}$"${\displaystyle }$: $=$ (${\displaystyle x}$ ,${\displaystyle 0}$ $)"$ $(\displaystyle$$x$\ :=$D$ ($x,0)$는 X에 $대한{\displaystyle }$ 규범적인 메트릭을 정의하고${\displaystyle }$"$display$ style${\displaystyle }$x$\cdot$$"$ 에 의해 유도됩니다$.$$}$

$($ ${\displaystyle X}$,${\displaystyle \Vert \cdot }$( ${\displaystyle (}$ ( ( ( ( ($xxxxxx$（ X , \$cdot$$\$$）$는 표준$$$$ 공간이고$,$${\displaystyle }$and $anddisplaydisplaydisplaydisplaydisplaydisplaydisplaydisplaydisplaydisplaydisplay$ and induced induced $induceddisplaydisplaydisplaydisplaydisplay{\displaystyle }$ $induced$ induced induced induced induced induced $induced$ induced induceddisplaydisplaydisplay$$ induced induced induced induced and and and and and and and and and and$$）는 X에서 유도되는$$$표준{\displaystyle }$ 토폴로지라고 합니다$.$\$displaystyle$X는$$ ${\displaystyle 」}$와 같습니다$.{$ $displaystyle$$\ tau$${\displaystyle \}}$$$D가$$$$ 변환 불변인^{[note 3]} $경우{\displaystyle }$${\displaystyle }$(${\displaystyle X}$,$\cdot \ )$는 (${\displaystyle X}$,$D$)$$\ $displaystyle ( X$ ,$$\ cdot \ )이$$ 완전한 메트릭 ^[9]공간인 경우에만${\displaystyle }$Banach 공간입니다$.$D$(\displaystyle$ D$)$가 변환 불변수가 아닌 $경우{\displaystyle }$,${\displaystyle }$(X$cdot \)${$displaystyle (X,\$cdot \)}은${\displaystyle }$Banach 공간이지만 (${\displaystyle X,}$ $)$ $displaystyle (X,D)}$은 완전한 메트릭^[10] 공간이 아닐 수 있습니다(예^{[note 6]}: 각주 참조).대조적으로, Klee,[11][12][노트 7]의 정리도 모두 계량화 가능 위상 벡터 공간에, 한다면, any[노트 8]X{X\displaystyle}에 X에서 표준 위상τ{\displaystyle \tau},{X\displaystyle,} 다음(X,‖ ⋅ ‖)을 유발하는 완전한 미터 D{D\displaystyle}이 존재하는 것을 의미하기 적용된다. {\displaystyle(X,\ \cdot $\ )$는 Banach 공간입니다.

프레셰 공간은 국소적으로 볼록한 위상 벡터 공간이며, 그 위상은 어떤 변환 불변 완전 메트릭에 의해 유도된다.모든 바나흐 공간은 프레셰 공간이지만 반대로는 아니다. 실제로 노름이 연속 함수인 프레셰 공간(실수열 $R{\mathbb{}}^{}$ ${\mathbb{}}^{}$ $\underset{}{}$ i$\underset{}{n\mathbb{}}$ $\underset{}{N}\mathbb{}$ R$${\$textyle \mathbb {R} ^{\mathbb {N} =$ \mathbb {N} \$mathbb}$ )도 존재한다$.$하지만, 모든 프레셰 공간의 위상은 규범의 일반화인 세미노름이라고 불리는 셀 수 있는 (필요하게 연속적인) 실제 값 지도 패밀리에 의해 유도된다.심지어 프레셰 공간은 계수 가능한 규범 패밀리에 의해 유도되는 위상을 가질 수 있지만(이러한 규범은 반드시 ^{[note 9][13]}연속적일 것이다), 그 위상은 어떤 하나의 규범에 의해 정의될 수 없기 때문에 바나흐/규범 공간이 될 수 없다.이러한 공간의 예로는 $Fréchet{\displaystyle {}^{}}$ 공간 C ${\displaystyle {}^{}}$ (${\displaystyle K}$)$$, \$displaystyle$ C$^{\infty$}($K)$가 있으며, 그 정의는 시험 함수 및 분포 공간에 관한 기사에서 찾을 수 있다.

완전한 표준 vs 완전한 토폴로지 벡터 공간

미터법 완전성 외에 또 다른 완전성의 개념이 있는데, 그것은 균일한 공간의 이론을 사용하는 완전 위상 벡터 공간(TVS) 또는 TV 완전성의 개념이다.특히 TV 완전성의 개념은 벡터 감산 및 벡터 공간이 부여되는 위상$(\displaystyle\tau$$)$에만 의존하는 표준 균일성이라고 불리는 고유한 변환 불변 균일성을 사용합니다. 따라서 TV 완전성의 개념은 유도된 어떤 규범과도 독립적입니다.$토폴로지{\displaystyle }$"\$displaystyle\tau"$ (측정할 수 없는 TV에도 적용됩니다)바나흐의 모든 공간은 완전한 TV입니다.또한, 노름 공간은 위상 벡터 공간으로서 완전한 경우에만 바나흐 공간이다(즉 완전함)이 위상 벡터 공간은 바나흐 공간이다.( ${\displaystyle X}$, ${\displaystyle )}$ )$${$display$ ( X , \ $tau )$이 측정 가능한 토폴로지 벡터 공간(예를 들어 표준유도 토폴로지 등$){\displaystyle }$인 ${\displaystyle \mathrm{경우}},{\displaystyle }$ ${\displaystyle (X}$,$$" ) { $displaystyle$(X , \ $tau$$$)}은 완전한 TV가 됩니다.이는 모든${\displaystyle \mathrm{Cauchy}}$ $({\displaystyle }$ )의 순서로 완전한 TV를 확인하는 것으로 충분합니다.le ( $X$, \ $tau )$는 (${\displaystyle X}$ , ${\displaystyle )}$ $)$ $\{{\displaystyle }$ $displaystyle$( $X$$$, \ $tau$)로 ${\displaystyle \mathrm{수렴}}$합니다$(즉$, 임의의 Cauchy nets의 일반적인 개념을 고려할 필요는 없습니다).

$({\displaystyle }$ ${\displaystyle X}$, ${\displaystyle )}$ )$${$display$( $X$, \$tau$) }가$$ 어떤 (아마도 알 수 없는) 노름에 의해 위상이 유도되는 토폴로지 벡터 공간인 ${\displaystyle \mathrm{경우}}$(이러한 공간은 노름이라고 불리며 원점의 경계 볼록한 근방을 갖는 것이 특징임$),{\displaystyle }$ ${\displaystyle (X}$ ,$$ $displaystyle$( $X$, \$tau$ )는 완전 벡토리컬이다.또는 X$(\displaystyle$ X$)$에 표준 $‖(\displaystyle \cdot \)$을 할당할 수 있는 $경우$에만 공백 또는 공백. 이 표준 or${\displaystyle (\backslash }$displaystyle X$)$은 X$(\displaystyle$$\tau$)에서 토폴로지 ${\displaystyle \{\backslash (\backslash }$$displaystyle$ \tau$)$를 유도하고 ($, \displaystyle$ \$cdot$\cdot $\)$를 바나흐$$ 공간으로${\displaystyle }$만듭니다.X{X\displaystyle}normable 있는 하우스 도르프 지역적으로 볼록 위상 벡터 공간 만일 강력한 이중 공간 Xb({\displaystyle X_{b}^{\prime}}은 바나흐 공간(Xb({\displaystyle X_{b}^{\prime}}은 공백이 있음을 나타낸다.에g${\displaystyle }$X의 $듀얼{\displaystyle }$ 스페이스$(\displaystyle$ X$$$).$ 토폴로지는 연속 듀얼 $스페이스{\displaystyle {}^{}}$ X의 듀얼 노름 유도 토폴로지의 일반화(\$displaystyle$ X$^{\prime$ $\})$입니다.자세한 내용은^{[note 10]} 이 각주를 참조하십시오.X$(\displaystyle$ X$)$가 측정 가능한 로컬 볼록 TV인 $경우{\displaystyle }$ X$(\displaystyle$ X$)$는 $(\$})가 Fréche-Uryson ^[15]공간인 $경우$에만 표준화할 수 있습니다.이는 로컬 볼록형 TV의 범주에서 바나흐 공간은 정확히 계량 가능한 동시에 계량 가능한 강한 이중 공간을 가진 완전한 공간임을 보여준다.

완료

모든 노름 공간은 바나흐 공간의 밀도 높은 벡터 부분 공간에 등각적으로 삽입될 수 있습니다. 여기서 바나흐 공간은 노름 공간의 완성이라고 불립니다.이 하우스도르프 완성은 등각 동형사상까지 독특하다.

보다 정확하게는 모든 표준 $공간{\displaystyle }$ X$,$ {\$displaystyle$X$}$에 대해 Banach $Y$와 $매핑{\displaystyle }$T:${\displaystyle X}$ ${\displaystyle \to }$(\$displaystyle$ T$:$$X\to$ Y$)$가 존재하며${\displaystyle ,}$ $T{\displaystyle }$${\displaystyle }${\$displaystyle$ T$(X)$는 등각 $매핑$이고 T$displaystyle$ T$($$X$)는 밀도가 높습니다$.$$Yle$Z는$$ 또 다른 Banach 공간입니다. X$(\displaystyle$X)에서 Z$(\displaystyle$ Z$)$의 조밀한 부분 집합으로 등각형이 $존재$하며 Z$(\displaystyle$ Z$)$는 Y$displaystyle$ Y$)$와 등각형이 됩니다$.$ 이 Banach $공간{\displaystyle }$Y(\$displaystyle$ Y$)$는 Haffus 스파의 완성입니다.$ce{\displaystyle }$ X$.$ {{$displaystyle$X$.}$$Y$의 $기본{\displaystyle }$ 메트릭 공간은${\displaystyle }$X의 $메트릭{\displaystyle }$ 완료와$$ 같습니다$.$ 벡터 공간 연산을$X$에서${\displaystyle }Y$로 확장한 $경우$ {{$displaystyle$ X$.}$의 완료는 때로 $표시$됩니다.$^.$$}$

일반론

선형 연산자, 동형사상

X$(\displaystyle$ X$)$와 Y$(\displaystyle$ Y$)$가 동일한 $그라운드{\displaystyle \mathbb{}}$ 필드${\displaystyle }$K$(\displaystyle \mathbb {K})$의 표준 공간인 $경우{\displaystyle }$$$ 모든 $연속{\displaystyle \mathbb{}}$ K$(\$ 선형$$ 맵 $T{\displaystyle :X}$ ${\displaystyle \to }$${\displaystyle (\backslash }$$displaystyle$ T$:X\to$Y)의$$ $집합$은${\displaystyle }$X(\to Y)로 $표시$됩니다.모든 선형 맵이 연속적인 것은 아닙니다.노름 $공간{\displaystyle }$ X에서$$다른$$ 노름 $공간$으로의 선형 매핑은${\displaystyle }$X의 닫힌 단위 볼에 경계가 있는 경우에만 연속됩니다$.$ $따라서$ 벡터 $공간{\displaystyle }$ B${\displaystyle (X,}$ $)$ {{$display style$$$B$(X, Y)}$는 연산자 노름이 주어질 수 있습니다.

Y$(\displaystyle$ Y$$$)$ Banach 공간의 경우 $공간{\displaystyle }$ B$(X,$는 이 기준에서 Banach 공간입니다.

X$({displaystyle$X})가$$ 바나흐 $공간$인 경우${\displaystyle }공간{\displaystyle }$ ${\displaystyle B(X}$) $=$ ${\displaystyle B(X,}$ X$)$ {$displaystyle B($X)=$B(X,$X)}는$$ 단치 바나흐 대수를 형성합니다. 곱셈 연산은 선형 맵의 합성에 의해 주어집니다.

$X({displaystyle$ X$}$와 Y$({displaystyle$Y})가 규범 공간인 $경우{\displaystyle }$, T$({displaystyle$ T$})$와 그 역${\displaystyle {}^{}}T{\displaystyle {}^{}}$ - $({$ T$^{-1})$이 $연속적$으로 선형 $분사{\displaystyle }$T:${\displaystyle X}$ ${\displaystyle \to }$(\$displaystyle$ T$:X\to$ Y$)$가 존재하는 경우에는 동형 규범 공간이다.X $또는$$Y{\displaystyle }$$($$또는$ 반사, 분리 가능 등)의$$ 두 $공간{\displaystyle }$ 중 하나가 완전하면 다른 공간도 완전해집니다.$또한$ T$$${\displaystyle \{\backslash }$$displaystyle$ T${\displaystyle \}}$가 등각인 경우 X {\$displaystyle$ X$}$와${\displaystyle }$Y {\${\displaystyle \mathrm{displaystyle}}$ Y$}$의 $두{\displaystyle }$ 개의 표준 $공간{\displaystyle }$X$(\displaystyle$X${\displaystyle )}$와 Y$(\displaystyle$ Y$)$는 등각형입니다$.$$$두 개의 동형 공간 X와$Y{\displaystyle }$ $사이$의 (\$displaystyle$$d(X,$Y)는$$등각 $공간$이 아닌 (\$displaystyle$ X$)$와 Y$(\displaystyle$ Y$)$의 차이를 나타냅니다$.$

연속 및 유계 선형 함수 및 세미노름

모든 연속 선형 연산자는 유계 선형 연산자이며, 규범화된 공간만 다루는 경우에는 그 반대도 참입니다.즉, 두 규범 공간 사이의 선형 연산자는 연속 함수인 경우에만 경계가 됩니다.따라서 특히 스칼라 필드($\$ $또는$ C$\$는 정규 공간이기 때문에 정규 공간상의 선형 함수는 연속 선형 함수인 경우에만 유계 선형 함수이다.이를 통해 (아래와 같은) 연속성 관련 결과를 Banach 공간에 적용할 수 있습니다.경계성은 규범 공간 사이의 선형 지도에 대한 연속성과 동일하지만, "경계"라는 용어는 바나흐 공간을 주로 다룰 때 더 일반적으로 사용됩니다.

만약 f:X→ R{\displaystyle f:X\to \mathbb{R}}은 만일 f{\displaystyle f}한결같이 모든 X{X\displaystyle}에 연속적입니다 then[16]f{\displaystyle f}발신지에서 연속은 저가 산적 함수(노르마, 선형적이기 때문 기능, 또는 실질적인 선형 기능과 같은),,, 또한 만약에 f(. 0 만일 f:X→는 경우에는 0, ∞){\displaystyle f:X\to -LSB- 0,\infty)}은 절대적인 값 연속적이다=0{\displaystyle f(0)=0} 다음 f{\displaystyle f}, X.{\displays의 만일{)∈ X:f())<1}{\displaystyle\와 같이{x\in X:f())<>1\}}은 개방되어 부분 집합은 일어나는 연속적입니다.Xtyle.}[16][노트 11]그리고 만일 이 현실의 부분의 사실은 매우 중요한 것은 그 Hahn–Banach 정리를 적용하기 위해 직선형 기능 f{\displaystyle f}리 ⁡ f{\displaystyle \operatorname{리}f}, ‖이 훌륭히 ⁡ f‖)‖ f‖}와 현실 부분f\ =\ f\{\displaystyle)\operatorname{리}이 훌륭히 기록한 연속적입니다. ⁡ f{\displ$aystyle \operatorname {Re}$f는 f$$, {$displaystyle$ f$,}$을 완전히 $결정$하므로 Han-Banach 정리가 종종 실제 선형 함수에 대해서만 언급됩니다.또한$X$의 $선형{\displaystyle }$ $함수{\displaystyle }$f는 ${\displaystyle seminorm}$f가$$ 연속인 경우에만 연속됩니다. seminorm $f$는 $seminorm{\displaystyle }$ f : X ${\displaystyle \to }$ ${\displaystyle R\mathbb{}}${\$displaystyle$ p$:X\to$ \$mathbb {R}$이(가) ${\displaystyle 존재}$하는 경우에만 연속 함수 f${\displaystyle }${\$displaystyle$f$}$이$(가$ f\$displaystyle$ f}$leq$ p$;$ 이 선형 $함수{\displaystyle }$ f$\displaystyle$f와$$ $seminorm$ p\$displaystyle$$$p를 포함하는 마지막 문장은 Han-Banach 정리의 많은 버전에서 볼 수 있다.

기본 개념

2개의 노름 공간의 $데카르트{\displaystyle }$곱 ${\displaystyle X}$× Y(\$displaystyle X\times$Y)는$$ 규범적으로 노름을 갖추고 있지 않다.그러나 다음과 같은 몇 가지 동등한 규범이 일반적으로 사용됩니다.^[17]

그리고 동형 규범 공간을 만들어냅니다.이 점에서${\displaystyle }제품{\displaystyle }$ ${\displaystyle X}$× Y(또는 $직합{\displaystyle }$ X$display$(\$displaystyle$X$\$$times$Y$))$는 두 가지 요인이 완전해야 완성됩니다.

M$({displaystyle$ M$})$이 표준 $공간{\displaystyle }$X의 닫힌 선형 부분 공간인 $경우,$ 몫 $공간{\displaystyle }$ X$({displaystyle$X/$M})$에는 자연 표준이 있습니다.

$X{\displaystyle }$$($${\displaystyle 스타일}$ X$)$가 ^[18]완성되었을 때 X$/$$M$$(스타일$ X$/$M)은$$ 바나치 공간입니다.X$({displaystyle$ X$}$에서 X${\displaystyle /M}$으로$,$ X$({displaystyle$$$X$/M$$})$를 $클래스{\displaystyle }$ x${\displaystyle +M}$으로$,$ X({$displaystyle$x$+$$M})$를 $전송$하는 비율 맵은 선형이며, M $= X,$ {$displaystyle$ M,}인 경우를 제외하고 $표준{\displaystyle \mathrm{}}$1,$${$displaystyle$1을 가집니다$$$.$

X$({$$displaystyle{\displaystyle }$$$X})의 닫힌 선형 부분공간$$ M$$$({displaystyle$ M$})$은 M({$displaystyle$$$M})이 투영경계 선형 $투영{\displaystyle }$ P$.({displaystyle$ P$:X\to$ M$})$의 범위인 $경우{\displaystyle }$$$X$({$$displaystyle$X})의 $보완{\displaystyle }$ 부분공간이라고 한다.$투영{\displaystyle }$P의 커널 M({$displaystyle$M$$$})$과${\displaystyle \ker }$ P$({displaystyle \ker$ P$)$의 $직접{\displaystyle }$ 합계에 ic$.\displaystyle$ P$.$

X$(\displaystyle$ X$)$와$$Y(\$displaystyle$ Y$)$가 Banach 공간이고 T$X, Y)$가$$T$(\displaystyle$$$T$)$라고^[18] 가정합니다.

여기서 첫 번째 맵 ${\$ { $displaystyle \pi }$는 몫 맵이고 두 번째 맵 ${\displaystyle {T1\mathrm{}}_{}}${ $displaystyle T_{1}$은 몫의 모든 $클래스{\displaystyle }$x + ${\displaystyle \ker }$†$$ { $displaystyle$ x + \ $ker$ T$$}를${\displaystyle }Y$의 $이미지{\displaystyle }$ T${\displaystyle (x}$로 전송합니다$.\displaystyle$ T$(x)$는 모두 동일하게 정의되어 있습니다$.$클래스는 같은 이미지를 가집니다.$매핑{\displaystyle {}_{}}$ $({$은 X${\displaystyle }$/ ${\displaystyle \ker t}$T({$displaystyle$ X$/\ker$T})에서 범위 $T{\displaystyle (X)}$ ,$$\$displaystyle$ T$(X)$로의 선형 분사이며, 이 값은 역방향으로 제한될 필요가 없습니다.

고전적 공간

바나흐 공간의 기본 examples[19]:Lp}과 그들의 특별한 케이스, 시퀀스 공간 p{\displaystyle\ell ^{p}ℓ}은 스칼라 시퀀스가 N{\displaystyle \mathbb{N}에 의해}인덱스로 구성되어 있어요;이들에는, 우주 ℓ 1{\displaystyle\ell ^{1}}나는 p{\displaystyle L^{p}spaces을 포함한다. 의bsolutely summable sequence와 space ${\displaystyle {22\mathrm{}}^{}}$$${\$displaystyle$\$ell$ $^{2}$},$$$space{\displaystyle {}_{}}$ c 0$${\$displaystyle c_{0$$$$},$ $scalarcti$의 $space{\displaystyle }$C ($K$$)$ (\$displaystyle$c_${\$$infty }$최대 노름을 갖춘 콤팩트 하우스도르프 $공간{\displaystyle }$ K$,$ \$displaystyle$ K$$$,$

그 Banach–Mazur 정리에 따르면, 매 바나흐 공간 같은 크기로. 일부 C(K)의 부분 공간에.{C(K)\displaystyle 동형이다.모든 분리 바나흐 공간 동안}[20]X,{X\displaystyle,}이 ℓ 1{\displaystyle\ell ^{1}의 폐쇄적인 부분 공간 M{M\displaystyle}은}가 X:)ℓ 1/M.{.$\displaystyle X:=\ell ^{1}/M.$$}$^[21]

임의의 힐베르트 공간은 바나흐 공간의 예로서 기능합니다.K ${\displaystyle =R\mathbb{}}$ ,$$ \$displaystyle \mathbb {K}$= \$mathbb {R}$ , \$mathbb {C}$ $위$의 Hilbert $공간{\displaystyle }$H {\$displaystyle$ H$}$는 형식의 규범에 대해 완전합니다.

어디에는 다음 조건을 만족시키는 첫 번째 인수에서 선형인 내부 곱입니다.

$예$를 들어 공간 $(\$L^{2})는$$ 힐베르트 공간 L2(\$display$ L$^{$2})는 힐베르트 공간입니다.

Hardy 공간, Sobolev 공간은 L $\$ L$^{p}$ 공간과 관련된$$ $추가{\displaystyle {}^{}}$ 구조를 가진 Banach 공간의 예입니다.그것들은 분석, 조화 분석, 편미분 방정식의 여러 분야에서 중요하다.

바나흐 대수

바나흐 대수는 K $=$ $위$의 바나흐 $A$$(\$$displaystyle$ \$mathbb$$${$K}$ =\$mathbb$${$$R$$}$$$${\displaystyle \}}$ 또는 $),$$$K $위$의 대수 구조$(\displaystyle$ \$mathbb${C$}$ )이며${\displaystyle ,}$ 곱${\displaystyle A}$는 $다음$과 같습니다${\displaystyle .}$$b)\mapsto$ab\$in$ A$}$는 연속적입니다.$A$${\displaystyle .\backslash \mathrm{displaystyle}}$ A.\$displaystyle$ a$.\$${\displaystyle \mathrm{displaystyle}}$ a.\$in$$$A.\displaystyle $a.\$displaystyle a.\displaystyle a$,b\in$ A$.\backslash {\displaystyle }$displaystyle a$.\$displaystyle A$.$에 ${\displaystyle \mathrm{대한}}$ 동등한 규범을 찾을 수 있습니다$.$

예

• Banach 공간${\displaystyle }C{\displaystyle }$ (${\displaystyle }$K )$$\ $displaystyle$C ($K$ )는$$ Banach 대수입니다.
• 디스크 $대수{\displaystyle }$ A$($ ${\displaystyle D\mathbf{}}$)(\$displaystyle$A(\$mathbf${D$})$는 오픈 유닛 디스크 D$d{\displaystyle }$ ${\displaystyle C\mathbb{}}$(\$displaystyle$ D$\subseteq \mathbb {C}})$에서 홀모형 함수와 닫힘 시 $연속함수{\displaystyle \stackrel{}{\mathbf{}}}$ D${\displaystyle \stackrel{}{}.}$ .$${\$displaystyle \mathbb {D}(D$})를 갖추고 있습니다.$mathbf {D}},}$ 디스크$$ $(\displaystyle$ A$(\mathbf {D})$는 C${\displaystyle (D\stackrel{}{\mathbf{}}))}$의 닫힌 하위 대수입니다. {\$displaystyle$ C$\left({\overline {D}}}}}\$$오른쪽).$$}$
• 위너 $대수{\displaystyle }$ A${\displaystyle (T\mathbf{}}$)(\$displaystyle$ A$(\mathbf {T})$는 절대 수렴 푸리에 급수를 갖는 단위 원$T{\displaystyle \mathbf{}}$(\$displaystyle \mathbf {T})$ 위의$$ 함수 대수입니다.$T${\displaystyle $\mathbf {T}}$ 위의$$ $함수$를 푸리에 계수의 시퀀스에 관련짓는 맵을 통해 이 대수는 바나흐 대수 ${\displaystyle {\delta \mathrm{}}^{}}$1 (${\displaystyle Z}$)$$, \$displaystyle \ell ^{1}(Z$)과$$ 동형이며$,$ 여기서 곱은 시퀀스의 컨볼루션이다.
• 모든 Banach $공간$에 대해$$X${\displaystyle }위$의 경계 선형 연산자의 $공간{\displaystyle }$ B$X)$ {$displaystyle$ B$(X$$)}$ {$displaystyle$ X$}$는 Banach 대수입니다.
• C*-algebra는 반직선 $변환{\displaystyle }$ a${\displaystyle {}^{}}$$displaystyle$ a$\$$map$ $to$ a$^{*})$를 갖는 복소수 Banach $대수{\displaystyle }$A(\$displaystyle A$\displaystyle $a\$ ${\displaystyle {to\mathrm{}}^{}}$ a$^{*})이다$.$$ 힐베르트 $공간{\displaystyle }$H(\$displaystyle$ H$)$의 경계 선형 연산자 $공간{\displaystyle }$ B$(H)$ (H$)$는 C*-대수의 기본 예입니다.Gelfand-Naimark 정리에서는 모든 C*-대수가 일부 $(H)$의 C*-하위 대수와 등각적으로 동형이라고 합니다$.$} 콤팩트$$ $하우스도르프{\displaystyle }$ 공간$K$(\$displaystyle$ K$$$)$ 상의 복잡한 연속 함수의 ${\displaystyle \mathrm{공간}}$C$(\displaystyle C(K$$))$는 $모든{\displaystyle }$ $f$(\$style$$$$f$)와 관련된 가환 C*-$대수$의 예입니다$.$$}$

이중 공간

X$(\displaystyle$ X$)$가 표준 공간이고 $(\$가 기본 필드(실수 또는 복소수)인 $경우{\displaystyle }$ 연속 이중 공간은 X$(\displaystyle$ X$)$에서 K$(\displaystyle \mathbb {K})$로 연속 선형 매핑된 공간입니다.이 ^[22]글에서 연속 쌍수의 $표기법$은 X ′${\displaystyle {\mathrm{}}^{}B}$ (${\displaystyle X}$ ,${\displaystyle K\mathbb{}}$) { $displaystyle$ X$^{\prime$ } $= B$ ($X,\mathbb {K}$ ) 입니다$$.K$(\$는 바나치 공간(절대값을 노름으로 사용)이므로 듀얼 $X{\displaystyle {}^{}}${\(\$displaystyle$ X$^{\prime})$는 모든 표준 공간 $X{\displaystyle }$ $.\displaystyle$ X$$}에 대해 바나치 공간입니다.

연속 선형 함수의 존재를 증명하는 주요 도구는 한-바나흐 정리이다.

특히, 노름 공간의 부분 공간상의 모든 연속 선형 함수는 ^[23]함수의 노름을 증가시키지 않고 공간 전체로 연속적으로 확장될 수 있다.중요한 특수한 경우는 다음과 같습니다.표준 $공간{\displaystyle }$X의 벡터$x{\displaystyle }$(\$displaystyle$$$X$)$마다 X$(\displaystyle$ X$)$에 $연속{\displaystyle }$ 선형 $함수{\displaystyle }$ f$(\displaystyle$ f$)$가 존재합니다.

x$(\displaystyle$ x$)$가 $(\$ 벡터와 같지 않은 $경우{\displaystyle }$ $함수{\displaystyle }$f(\$displaystyle$ f$)$에는 표준이 있어야 하며 x$(\displaystyle$ x$)$의 $표준{\displaystyle }$ 함수라고 합니다.

한-바나흐 분리 정리에 따르면, 실제 바나흐 공간에서 비어있지 않은 두 개의 볼록한 집합은 닫힌 아핀 초평면으로 분리될 수 있다.오픈 볼록 세트는 엄밀하게 하이퍼플레인 한쪽에 있습니다.두 번째 볼록 세트는 다른 쪽에 있지만 하이퍼플레인에 ^[24]닿을 수 있습니다.

Banach $공간{\displaystyle }$X의 부분 $집합{\displaystyle }$S$(\$$displaystyle$ X$)$는 S$(\displaystyle$ S$)$의 $선형{\displaystyle }$ 범위가${\displaystyle }$X(\$displaystyle$ X$)$로 $조밀$하면 합계가 되고, S$(\$$displaystyle$ S$)$는 S$(\displaystyle$ X)에서 연속 선형 함수만 소멸하면$합계$가 됩니다$.$$ystyle$S는$$ ${\displaystyle \mathbf$ {0$}$ 함수입니다$$. 이 등가는 Han-Banach 정리에 따릅니다.

두 문을 닫선형 subspaces M{M\displaystyle}과 N,{\displaystyle N,}의 만약 X{X\displaystyle}직접적인 합 다음 이중 X′}X의{X\displaystyle}M{M\displaystyle}과 N의 duals의 직접적인 합.{N\displaystyle}[25]만약 동형은{\displaystyle X^{\prime}. $M({displaystyle$ M})은${\displaystyle }X$의 닫힌 선형 부분 공간이며$,$ $듀얼$의 M({$displaystyle$$$M$})$과 직교할 수 있습니다.

$직교{\displaystyle {}^{}}$ M ${\$ {\$displaystyle$ M$^{\bot$}}은$$ 듀얼의 닫힌 선형 부분 공간입니다.$M$의$$쌍대({$displaystyle$ M$})$는 X${\displaystyle }$${\displaystyle /}$ / ${\displaystyle M{}^{}}$$$${\displaystyle {}^{}}$ . ${\displaystyle$ X$'/M^{\$$bot}$ )는 M ${\displaystyle {}^{}}$ .$${\$displaystyle$ M$^{\bot}$과 동형입니다.

분리 가능한 Banach 공간의 듀얼은 분리할 수 없습니다.

X-$(\$ X$')$를 분리할 수 있는 $경우{\displaystyle {}^{}}$ 위의 종합성 기준을 사용하여${\displaystyle }$X.\$displaystyle$ X에 $카운트$ 가능한 총 서브셋이 존재함을 증명할 수 있습니다$.$

취약한 토폴로지

Banach $공간{\displaystyle }$ X$({displaystyle$X})의 약한 토폴로지는 X$({displaystyle$X})에서 가장 거친 토폴로지로 연속된 듀얼 $공간{\displaystyle {}^{}}$ X$({$ X$^{\prime$}}}) 내의 모든 $요소{\displaystyle {}^{}}$x({$displaystyle$x^{\prime$})$가 연속됩니다.따라서 표준 토폴로지는 약한 토폴로지보다 미세합니다.한-바나흐 분리 정리에 따르면 약한 위상은 하우스도르프이며, 바나흐 공간의 노름 닫힌 볼록 부분 집합도 ^[28]약하게 닫혀 있다.2개의 Banach $공간{\displaystyle }$X({$displaystyle$X})와$$Y({$displaystyle$Y}) 사이의 표준 연속 선형 맵도 약하게 연속됩니다. 즉, X$({displaystyle$X})의$$ $약한{\displaystyle }$ 토폴로지부터 Y$({displaystyle$ Y})까지 연속적입니다$.$

X$(\displaystyle$ X$)$가 무한 차원일 $경우{\displaystyle }$ 연속적이지 않은 선형 맵이 존재합니다.X$(\displaystyle$ X$)$에서 기본 $필드{\displaystyle \mathbb{}}$K(\$displaystyle \mathbb {K})$에 이르는 모든 선형 맵의 $공간{\displaystyle {}^{}}$ X$(\$$displaystyle$ X$^{*})$도${\displaystyle {}^{}}$토폴로지에서 X$(\$ X$^{*})$와 구별하기 위해 대수적 이중 공간이라고 $합니다{\displaystyle {}^{}}$$.$$X$$($디스플레이 스타일 X)는 약한 토폴로지보다 미세하고 기능 해석에 많이 사용되지 않습니다.

듀얼 $스페이스{\displaystyle {}^{}}$ X ${\displaystyle {}^{}}$、 \ $display style$ X^ { \ $prime }$ 에는$$ 약한 토폴로지 X ${\displaystyle {}^{}}$ $、$ \ $display style$ X^ { \ $prime }$보다 약한 토폴로지가 있습니다.$이$ 토폴로지는 X에서 가장 조잡한 토폴로지이며$,$ 모든 평가는 X $x{\displaystyle {}^{}{}^{}{、\mathrm{}}^{}}$、 \ $display$ $x$$$^ { \ $prime$ $}$ \ $in$ X ^ { \ $prime$ $}$ \ mapstyle X$$$$${\displaystyle {、\mathrm{}}^{}}$ $X{\displaystyle }$、 \ $style$ ）$$。그것의 중요성은 바나흐-알라오글루 정리로부터 온다.

바나흐-알라오글루 정리는 콤팩트 하우스도르프 공간의 무한곱에 대한 티코노프의 정리를 사용하여 증명될 수 있다.X$(\displaystyle$ X$)$가 분리 가능한 경우 $듀얼$의 $유닛볼{\displaystyle {}^{}}$ B$(\$ B$^{\prime$})는$$ weak* ^[30]토폴로지의 미터화 가능 콤팩트입니다.

이중 공간의 예

${\displaystyle {c\mathrm{}}_{}}$ 0$({$$\})$의 쌍은 c${\displaystyle {}_{}{0}_{}}$의$모든{\displaystyle {}_{}}$ 경계 선형 $함수{\displaystyle }$ f$({$$displaystyle$$$$c_{0})$에 대해 ${\displaystyle {1\mathrm{}}^{}}$1$({$ y$=\lefty_{n})$과 등각적으로 동형입니다.그거

${\displaystyle {1\mathrm{}}^{}}$1의 쌍대({$displaystyle \ell$^{$1})$는 $({$과 등형이다.르베그 $공간{\displaystyle {}^{}}$ ${\displaystyle L^{}}$p (${\displaystyle }$[${\displaystyle }$0 , ${\displaystyle 1]}$ )$$（ { $displaystyle L$^{p } ( [$$$0$ , $1$] $)$의 쌍대는 1µp< 1 \ $displaystyle$1 \$leq$$$p \ $infty }$ 및${\displaystyle }1{\displaystyle \frac{\mathrm{}}{}}$ + ${\displaystyle 1\frac{\mathrm{}}{}\mathrm{}=}$ ${\displaystyle \mathrm{1일}}$ 때 ${\displaystyle L^{}}$q${\displaystyle }$( [${\displaystyle 0}$ ,$1$ )와 등각적으로 동형이다.$}$

Hilbert $공간{\displaystyle }$H의 모든$$ $벡터{\displaystyle }$y$(\$$displaystyle$ y$),$ \$displaystyle$H에 대한$$ 매핑

는${\displaystyle }$연속 선형 $함수{\displaystyle {}_{}}$ ${\displaystyle {fy}_{}}${\$displaystyle$ h$.}$ 를 정의합니다$$.Riesz 표현정리는 H$.\displaystyle$ H$.$의 모든 연속 선형 함수는 H$.displaystyle$ $f_{y}$ 형식의$$ 고유 정의된 $벡터{\displaystyle }$y$.\$$displaystyle$f_$$${y}$ 입니다.$playstyle$ H$.}$ ${\displaystyle \mathrm{매핑<img\; alt="H."\; aria-hidden="true"\; class="mwe-math-fallback-image-inline"\; src="https://wikimedia.org/api/rest\_v1/media/math/render/svg/8933ae7244305ae7824aa18e077d1cf946e2ee9d"\; style="vertical-align:\; -0.338ex;\; width:2.71ex;\; height:2.176ex;">}}$ $y{\displaystyle }$ ${\displaystyle \to }$ ${\displaystyle f_{}}$ y$$\ $displaystyle$ y$\in$ H$\to f_y}$ 는$$ H$${\$displaystyle$H } 에서 $듀얼{\displaystyle {}^{}}$ H ${\displaystyle .}$ 에 대한 반직선 등각분사입니다$.\$ $displaystyle$ H$'}$ 스칼라가 실재할 경우 이 맵은 등각형상입니다.

K$(\displaystyle$ K$)$가 콤팩트 하우스도르프 위상 공간일 $때{\displaystyle }$, C$K)$의 $듀얼{\displaystyle }$M$(\$$displaystyle$M$(K$$))$은 부르바키(^[31]Bourbaki)의 의미에서 라돈 측정 공간이다.M(K$)$의 부분 $집합{\displaystyle }$ P$)$ {$displaystyle$$$P$($$K)}$는 질량 1${\displaystyle (}$확률 측도)의 음이 아닌 측정값으로 구성되며$$, M$(K)$의 단위 볼(K)의 볼록 w* 닫힌 부분 집합이다.} ${\displaystyle \mathrm{P<img\; alt="\{\backslash displaystyle\; M(K).\}"\; aria-hidden="true"\; class="mwe-math-fallback-image-inline"\; src="https://wikimedia.org/api/rest\_v1/media/math/render/svg/6dbe48265ef0d69ae5c30c394a61b2a4dd3aa4b9"\; style="vertical-align:\; -0.838ex;\; width:6.964ex;\; height:2.843ex;">}}$( $K$) { $displaystyle P$ ($$K$$ ) } $、$ $K{\displaystyle }$ . { $displaystyle$ K$}$의 Dirac $측정치$입니다.W* 토폴로지를 탑재한$K$의 Dirac $측정치$ 집합은$$K . { $displaystyle$ K$$}와 $같은{\displaystyle }$ $형태$입니다$.$

결과는 Amir와^[35] Cambern에 의해^[34] C${\displaystyle (\backslash }displaystyle$ C($K)$와 C($L)$ $사이$의 승수 바나흐-마주르 거리가2 인 경우로 확장되었으며, 거리가 $= 2(\$$displaystyle C($$L)$일 경우 정리가 더 이상 참이 아닙니다$.$$}$^[36]

가환 $Banach{\displaystyle }$ 대수${\displaystyle }$C (${\displaystyle K}$) ,$$\ $displaystyle$C ( $K$) , \ $displaystyle$ K$,$\ displaystyle K , \ }에서 최대 이상은 정확히${\displaystyle }$K에 대한 Dirac 측정의 핵심입니다.

보다 일반적으로, 겔판드-마주르 정리에 의해, 단치환 바나흐 대수의 최대 이상은 집합으로서뿐만 아니라 위상 공간으로서, 즉 전자는 선체-커널 위상을, 후자는 w*-위상을 갖는 특성으로 식별될 수 있다.이 식별에서 최대 이상적인 공간은 $이중{\displaystyle {}^{}}$ A ${\displaystyle .}$ .$$\ $displaystyle$ A$'$에서 단위 볼의 w* 콤팩트 서브셋으로 볼 수 있습니다.$}$

콤팩트한 $하우스도르프{\displaystyle }$ 공간${\displaystyle }$K에 대해 모든 단치환 Banach 대수가 C$)\displaystyle$C($K)$ $형식$인 것은 아닙니다$.$ 단,${\displaystyle 이}$문장은$$C(K)\displaystyle C$($K)\$displaystyle$ C($K$)\displaystyle Bras의 작은 카테고리에 C(K)를 배치됩니다.가환 C*-대수에 대한 Gelfand의 표현정리에 따르면 모든 가환 단수 C*-$대수{\displaystyle }$ A(\$displaystyle$ A$)$는 C$(\displaystyle$ C$(K)}$ 공간과 ^[37]$등형$이다.여기서 하우스도르프 $콤팩트{\displaystyle }$ 공간$$K(\$displaystyle$K)는$$ C*-대게브라 맥락에서$$A(\$displaystyle$ A$$$)$의 스펙트럼이라고도 불리는 최대 이상 공간입니다.

쌍방향

X$(\displaystyle$X)가$$ 표준 공간인 $경우{\displaystyle }$, $듀얼{\displaystyle {}^{}}$ X$(\$ X$)$의 (연속) $듀얼{\displaystyle {}^{}}$ X${\displaystyle {(\backslash }^{}}$$style$ X$)$를 쌍방향 또는 X$displaystyle$ X$)$의 두 번째 듀얼이라고 합니다$.$ 모든$$ 표준 $공간{\displaystyle }$X$(\displaystyle$ X$)$에는 자연 지도가 있습니다.

This defines ${\displaystyle F_{X}(x)}$ as a continuous linear functional on ${\displaystyle X^{\prime },}$ that is, an element of ${\displaystyle X^{\prime \prime }.}$ The map ${\displaystyle F_{X}:x\to F_{X}(x)}$ is a linear map from ${\displaystyle X}$ to ${\displaystyle X^{\prime \prime }.}$ As a consequence of the existence of a norming functional ${\displaystyle f}$ for every ${\displaystyle x\in X,}$ this map ${\displaystyle F_{X}}$ is isometric, thus injective.

For example, the dual of ${\displaystyle X=c_{0}}$ is identified with ${\displaystyle \ell ^{1},}$ and the dual of ${\displaystyle \ell ^{1}}$ is identified with ${\displaystyle \ell ^{\infty },}$ the space of bounded scalar sequences. Under these identifications, ${\displaystyle F_{X}}$ is the inclusion map from ${\displaystyle c_{0}}$ to ${\displaystyle \ell ^{\infty }.}$ It is indeed isometric, but not onto.

If ${\displaystyle F_{X}}$ is surjective, then the normed space ${\displaystyle X}$ is called reflexive (see below). Being the dual of a normed space, the bidual ${\displaystyle X''}$ is complete, therefore, every reflexive normed space is a Banach space.

Using the isometric embedding ${\displaystyle F_{X},}$ it is customary to consider a normed space ${\displaystyle X}$ as a subset of its bidual. When ${\displaystyle X}$ is a Banach space, it is viewed as a closed linear subspace of ${\displaystyle X^{\prime \prime }.}$ If ${\displaystyle X}$ is not reflexive, the unit ball of ${\displaystyle X}$ is a proper subset of the unit ball of ${\displaystyle X^{\prime \prime }.}$ The Goldstine theorem states that the unit ball of a normed space is weakly*-dense in the unit ball of the bidual. In other words, for every ${\displaystyle x''}$ in the bidual, there exists a net ${\displaystyle \left(x_{i}\right)_{i\in I}}$ in ${\displaystyle X}$ so that

The net may be replaced by a weakly*-convergent sequence when the dual ${\displaystyle X'}$ is separable. On the other hand, elements of the bidual of ${\displaystyle \ell ^{1}}$ that are not in ${\displaystyle \ell ^{1}}$ cannot be weak*-limit of sequences in ${\displaystyle \ell ^{1},}$ since ${\displaystyle \ell ^{1}}$ is weakly sequentially complete.

Banach's theorems

Here are the main general results about Banach spaces that go back to the time of Banach's book (Banach (1932)) and are related to the Baire category theorem. According to this theorem, a complete metric space (such as a Banach space, a Fréchet space or an F-space) cannot be equal to a union of countably many closed subsets with empty interiors. Therefore, a Banach space cannot be the union of countably many closed subspaces, unless it is already equal to one of them; a Banach space with a countable Hamel basis is finite-dimensional.

The Banach–Steinhaus theorem is not limited to Banach spaces. It can be extended for example to the case where ${\displaystyle X}$ is a Fréchet space, provided the conclusion is modified as follows: under the same hypothesis, there exists a neighborhood ${\displaystyle U}$ of ${\displaystyle \mathbf {0} }$ in ${\displaystyle X}$ such that all ${\displaystyle T}$ in ${\displaystyle F}$ are uniformly bounded on ${\displaystyle U,}$

This result is a direct consequence of the preceding Banach isomorphism theorem and of the canonical factorization of bounded linear maps.

This is another consequence of Banach's isomorphism theorem, applied to the continuous bijection from ${\displaystyle M_{1}\oplus \cdots \oplus M_{n}}$ onto ${\displaystyle X}$ sending ${\displaystyle m_{1},\cdots ,m_{n}}$ to the sum ${\displaystyle m_{1}+\cdots +m_{n}.}$

Reflexivity

The normed space ${\displaystyle X}$ is called reflexive when the natural map

is surjective. Reflexive normed spaces are Banach spaces.

This is a consequence of the Hahn–Banach theorem. Further, by the open mapping theorem, if there is a bounded linear operator from the Banach space ${\displaystyle X}$ onto the Banach space ${\displaystyle Y,}$ then ${\displaystyle Y}$ is reflexive.

Indeed, if the dual ${\displaystyle Y^{\prime }}$ of a Banach space ${\displaystyle Y}$ is separable, then ${\displaystyle Y}$ is separable. If ${\displaystyle X}$ is reflexive and separable, then the dual of ${\displaystyle X^{\prime }}$ is separable, so ${\displaystyle X^{\prime }}$ is separable.

Hilbert spaces are reflexive. The ${\displaystyle L^{p}}$ spaces are reflexive when ${\displaystyle 1<p<\infty .}$ More generally, uniformly convex spaces are reflexive, by the Milman–Pettis theorem. The spaces ${\displaystyle c_{0},\ell ^{1},L^{1}([0,1]),C([0,1])}$ are not reflexive. In these examples of non-reflexive spaces ${\displaystyle X,}$ the bidual ${\displaystyle X''}$ is "much larger" than ${\displaystyle X.}$ Namely, under the natural isometric embedding of ${\displaystyle X}$ into ${\displaystyle X''}$ given by the Hahn–Banach theorem, the quotient ${\displaystyle X^{\prime \prime }/X}$ is infinite-dimensional, and even nonseparable. However, Robert C. James has constructed an example^[38] of a non-reflexive space, usually called "the James space" and denoted by ${\displaystyle J,}$^[39] such that the quotient ${\displaystyle J^{\prime \prime }/J}$ is one-dimensional. Furthermore, this space ${\displaystyle J}$ is isometrically isomorphic to its bidual.

When ${\displaystyle X}$ is reflexive, it follows that all closed and bounded convex subsets of ${\displaystyle X}$ are weakly compact. In a Hilbert space ${\displaystyle H,}$ the weak compactness of the unit ball is very often used in the following way: every bounded sequence in ${\displaystyle H}$ has weakly convergent subsequences.

Weak compactness of the unit ball provides a tool for finding solutions in reflexive spaces to certain optimization problems. For example, every convex continuous function on the unit ball ${\displaystyle B}$ of a reflexive space attains its minimum at some point in ${\displaystyle B.}$

As a special case of the preceding result, when ${\displaystyle X}$ is a reflexive space over ${\displaystyle \mathbb {R} ,}$ every continuous linear functional ${\displaystyle f}$ in ${\displaystyle X^{\prime }}$ attains its maximum ${\displaystyle \ f\ }$ on the unit ball of ${\displaystyle X.}$ The following theorem of Robert C. James provides a converse statement.

The theorem can be extended to give a characterization of weakly compact convex sets.

On every non-reflexive Banach space ${\displaystyle X,}$ there exist continuous linear functionals that are not norm-attaining. However, the Bishop–Phelps theorem^[40] states that norm-attaining functionals are norm dense in the dual ${\displaystyle X^{\prime }}$ of ${\displaystyle X.}$

Weak convergences of sequences

A sequence ${\displaystyle \left\{x_{n}\right\}}$ in a Banach space ${\displaystyle X}$ is weakly convergent to a vector ${\displaystyle x\in X}$ if ${\displaystyle \left\{f\left(x_{n}\right)\right\}}$ converges to ${\displaystyle f(x)}$ for every continuous linear functional ${\displaystyle f}$ in the dual ${\displaystyle X^{\prime }.}$ The sequence ${\displaystyle \left\{x_{n}\right\}}$ is a weakly Cauchy sequence if ${\displaystyle \left\{f\left(x_{n}\right)\right\}}$ converges to a scalar limit ${\displaystyle L(f),,}$ for every ${\displaystyle f}$ in ${\displaystyle X^{\prime }.}$ A sequence ${\displaystyle \left\{f_{n}\right\}}$ in the dual ${\displaystyle X^{\prime }}$ is weakly* convergent to a functional ${\displaystyle f\in X^{\prime }}$ if ${\displaystyle f_{n}(x)}$ converges to ${\displaystyle f(x)}$ for every ${\displaystyle x}$ in ${\displaystyle X.}$ Weakly Cauchy sequences, weakly convergent and weakly* convergent sequences are norm bounded, as a consequence of the Banach–Steinhaus theorem.

When the sequence ${\displaystyle \left\{x_{n}\right\}}$ in ${\displaystyle X}$ is a weakly Cauchy sequence, the limit ${\displaystyle L}$ above defines a bounded linear functional on the dual ${\displaystyle X^{\prime },}$ that is, an element ${\displaystyle L}$ of the bidual of ${\displaystyle X,}$ and ${\displaystyle L}$ is the limit of ${\displaystyle \left\{x_{n}\right\}}$ in the weak*-topology of the bidual. The Banach space ${\displaystyle X}$ is weakly sequentially complete if every weakly Cauchy sequence is weakly convergent in ${\displaystyle X.}$ It follows from the preceding discussion that reflexive spaces are weakly sequentially complete.

An orthonormal sequence in a Hilbert space is a simple example of a weakly convergent sequence, with limit equal to the ${\displaystyle \mathbf {0} }$ vector. The unit vector basis of ${\displaystyle \ell ^{p}}$ for ${\displaystyle 1<p<\infty ,}$ or of ${\displaystyle c_{0},}$ is another example of a weakly null sequence, that is, a sequence that converges weakly to ${\displaystyle \mathbf {0} .}$ For every weakly null sequence in a Banach space, there exists a sequence of convex combinations of vectors from the given sequence that is norm-converging to ${\displaystyle \mathbf {0} .}$^[42]

The unit vector basis of ${\displaystyle \ell ^{1}}$ is not weakly Cauchy. Weakly Cauchy sequences in ${\displaystyle \ell ^{1}}$ are weakly convergent, since ${\displaystyle L^{1}}$-spaces are weakly sequentially complete. Actually, weakly convergent sequences in ${\displaystyle \ell ^{1}}$ are norm convergent.^[43] This means that ${\displaystyle \ell ^{1}}$ satisfies Schur's property.

Results involving the ${\displaystyle \ell ^{1}}$ basis

Weakly Cauchy sequences and the ${\displaystyle \ell ^{1}}$ basis are the opposite cases of the dichotomy established in the following deep result of H. P. Rosenthal.^[44]

A complement to this result is due to Odell and Rosenthal (1975).

By the Goldstine theorem, every element of the unit ball ${\displaystyle B^{\prime \prime }}$ of ${\displaystyle X^{\prime \prime }}$ is weak*-limit of a net in the unit ball of ${\displaystyle X.}$ When ${\displaystyle X}$ does not contain ${\displaystyle \ell ^{1},}$ every element of ${\displaystyle B^{\prime \prime }}$ is weak*-limit of a sequence in the unit ball of ${\displaystyle X.}$^[47]

When the Banach space ${\displaystyle X}$ is separable, the unit ball of the dual ${\displaystyle X^{\prime },}$ equipped with the weak*-topology, is a metrizable compact space ${\displaystyle K,}$^[30] and every element ${\displaystyle x^{\prime \prime }}$ in the bidual ${\displaystyle X^{\prime \prime }}$ defines a bounded function on ${\displaystyle K}$:

This function is continuous for the compact topology of ${\displaystyle K}$ if and only if ${\displaystyle x^{\prime \prime }}$ is actually in ${\displaystyle X,}$ considered as subset of ${\displaystyle X^{\prime \prime }.}$ Assume in addition for the rest of the paragraph that ${\displaystyle X}$ does not contain ${\displaystyle \ell ^{1}.}$ By the preceding result of Odell and Rosenthal, the function ${\displaystyle x^{\prime \prime }}$ is the pointwise limit on ${\displaystyle K}$ of a sequence ${\displaystyle \left\{x_{n}\right\}\subseteq X}$ of continuous functions on ${\displaystyle K,}$ it is therefore a first Baire class function on ${\displaystyle K.}$ The unit ball of the bidual is a pointwise compact subset of the first Baire class on ${\displaystyle K.}$^[48]

Sequences, weak and weak* compactness

When ${\displaystyle X}$ is separable, the unit ball of the dual is weak*-compact by the Banach–Alaoglu theorem and metrizable for the weak* topology,^[30] hence every bounded sequence in the dual has weakly* convergent subsequences. This applies to separable reflexive spaces, but more is true in this case, as stated below.

The weak topology of a Banach space ${\displaystyle X}$ is metrizable if and only if ${\displaystyle X}$ is finite-dimensional.^[49] If the dual ${\displaystyle X'}$ is separable, the weak topology of the unit ball of ${\displaystyle X}$ is metrizable. This applies in particular to separable reflexive Banach spaces. Although the weak topology of the unit ball is not metrizable in general, one can characterize weak compactness using sequences.

A Banach space ${\displaystyle X}$ is reflexive if and only if each bounded sequence in ${\displaystyle X}$ has a weakly convergent subsequence.^[51]

A weakly compact subset ${\displaystyle A}$ in ${\displaystyle \ell ^{1}}$ is norm-compact. Indeed, every sequence in ${\displaystyle A}$ has weakly convergent subsequences by Eberlein–Šmulian, that are norm convergent by the Schur property of ${\displaystyle \ell ^{1}.}$

Schauder bases

A Schauder basis in a Banach space ${\displaystyle X}$ is a sequence ${\displaystyle \left\{e_{n}\right\}_{n\geq 0}}$ of vectors in ${\displaystyle X}$ with the property that for every vector ${\displaystyle x\in X,}$ there exist uniquely defined scalars ${\displaystyle \left\{x_{n}\right\}_{n\geq 0}}$ depending on ${\displaystyle x,}$ such that

Banach spaces with a Schauder basis are necessarily separable, because the countable set of finite linear combinations with rational coefficients (say) is dense.

It follows from the Banach–Steinhaus theorem that the linear mappings ${\displaystyle \left\{P_{n}\right\}}$ are uniformly bounded by some constant ${\displaystyle C.}$ Let ${\displaystyle \left\{e_{n}^{*}\right\}}$ denote the coordinate functionals which assign to every ${\displaystyle x}$ in ${\displaystyle X}$ the coordinate ${\displaystyle x_{n}}$ of ${\displaystyle x}$ in the above expansion. They are called biorthogonal functionals. When the basis vectors have norm ${\displaystyle 1,}$ the coordinate functionals ${\displaystyle \left\{e_{n}^{*}\right\}}$ have norm ${\displaystyle \,\leq 2C}$ in the dual of ${\displaystyle X.}$

Most classical separable spaces have explicit bases. The Haar system ${\displaystyle \left\{h_{n}\right\}}$ is a basis for ${\displaystyle L^{p}([0,1]),1\leq p<\infty .}$ The trigonometric system is a basis in ${\displaystyle L^{p}(\mathbf {T} )}$ when ${\displaystyle 1<p<\infty .}$ The Schauder system is a basis in the space ${\displaystyle C([0,1]).}$^[52] The question of whether the disk algebra ${\displaystyle A(\mathbf {D} )}$ has a basis^[53] remained open for more than forty years, until Bočkarev showed in 1974 that ${\displaystyle A(\mathbf {D} )}$ admits a basis constructed from the Franklin system.^[54]

Since every vector ${\displaystyle x}$ in a Banach space ${\displaystyle X}$ with a basis is the limit of ${\displaystyle P_{n}(x),}$ with ${\displaystyle P_{n}}$ of finite rank and uniformly bounded, the space ${\displaystyle X}$ satisfies the bounded approximation property. The first example by Enflo of a space failing the approximation property was at the same time the first example of a separable Banach space without a Schauder basis.^[55]

Robert C. James characterized reflexivity in Banach spaces with a basis: the space ${\displaystyle X}$ with a Schauder basis is reflexive if and only if the basis is both shrinking and boundedly complete.^[56] In this case, the biorthogonal functionals form a basis of the dual of ${\displaystyle X.}$

Tensor product

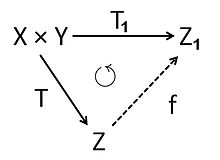
Let ${\displaystyle X}$ and ${\displaystyle Y}$ be two ${\displaystyle \mathbb {K} }$-vector spaces. The tensor product ${\displaystyle X\otimes Y}$ of ${\displaystyle X}$ and ${\displaystyle Y}$ is a ${\displaystyle \mathbb {K} }$-vector space ${\displaystyle Z}$ with a bilinear mapping ${\displaystyle T:X\times Y\to Z}$ which has the following universal property:

If ${\displaystyle T_{1}:X\times Y\to Z_{1}}$ is any bilinear mapping into a ${\displaystyle \mathbb {K} }$-vector space ${\displaystyle Z_{1},}$ then there exists a unique linear mapping ${\displaystyle f:Z\to Z_{1}}$ such that ${\displaystyle T_{1}=f\circ T.}$

The image under ${\displaystyle T}$ of a couple ${\displaystyle (x,y)}$ in ${\displaystyle X\times Y}$ is denoted by ${\displaystyle x\otimes y,}$ and called a simple tensor. Every element ${\displaystyle z}$ in ${\displaystyle X\otimes Y}$ is a finite sum of such simple tensors.

There are various norms that can be placed on the tensor product of the underlying vector spaces, amongst others the projective cross norm and injective cross norm introduced by A. Grothendieck in 1955.^[57]

In general, the tensor product of complete spaces is not complete again. When working with Banach spaces, it is customary to say that the projective tensor product^[58] of two Banach spaces ${\displaystyle X}$ and ${\displaystyle Y}$ is the completion ${\displaystyle X{\widehat {\otimes }}_{\pi }Y}$ of the algebraic tensor product ${\displaystyle X\otimes Y}$ equipped with the projective tensor norm, and similarly for the injective tensor product^[59] ${\displaystyle X{\widehat {\otimes }}_{\varepsilon }Y.}$ Grothendieck proved in particular that^[60]

where ${\displaystyle K}$ is a compact Hausdorff space, ${\displaystyle C(K,Y)}$ the Banach space of continuous functions from ${\displaystyle K}$ to ${\displaystyle Y}$ and ${\displaystyle L^{1}([0,1],Y)}$ the space of Bochner-measurable and integrable functions from ${\displaystyle [0,1]}$ to ${\displaystyle Y,}$ and where the isomorphisms are isometric. The two isomorphisms above are the respective extensions of the map sending the tensor ${\displaystyle f\otimes y}$ to the vector-valued function ${\displaystyle s\in K\to f(s)y\in Y.}$

Tensor products and the approximation property

Let ${\displaystyle X}$ be a Banach space. The tensor product ${\displaystyle X'{\widehat {\otimes }}_{\varepsilon }X}$ is identified isometrically with the closure in ${\displaystyle B(X)}$ of the set of finite rank operators. When ${\displaystyle X}$ has the approximation property, this closure coincides with the space of compact operators on ${\displaystyle X.}$

For every Banach space ${\displaystyle Y,}$ there is a natural norm ${\displaystyle 1}$ linear map

obtained by extending the identity map of the algebraic tensor product. Grothendieck related the approximation problem to the question of whether this map is one-to-one when ${\displaystyle Y}$ is the dual of ${\displaystyle X.}$ Precisely, for every Banach space ${\displaystyle X,}$ the map is one-to-one if and only if ${\displaystyle X}$ has the approximation property.^[61]

Grothendieck conjectured that ${\displaystyle X{\widehat {\otimes }}_{\pi }Y}$ and ${\displaystyle X{\widehat {\otimes }}_{\varepsilon }Y}$ must be different whenever ${\displaystyle X}$ and ${\displaystyle Y}$ are infinite-dimensional Banach spaces. This was disproved by Gilles Pisier in 1983.^[62] Pisier constructed an infinite-dimensional Banach space ${\displaystyle X}$ such that ${\displaystyle X{\widehat {\otimes }}_{\pi }X}$ and ${\displaystyle X{\widehat {\otimes }}_{\varepsilon }X}$ are equal. Furthermore, just as Enflo's example, this space ${\displaystyle X}$ is a "hand-made" space that fails to have the approximation property. On the other hand, Szankowski proved that the classical space ${\displaystyle B\left(\ell ^{2}\right)}$ does not have the approximation property.^[63]

Some classification results

Characterizations of Hilbert space among Banach spaces

A necessary and sufficient condition for the norm of a Banach space ${\displaystyle X}$ to be associated to an inner product is the parallelogram identity:

It follows, for example, that the Lebesgue space ${\displaystyle L^{p}([0,1])}$ is a Hilbert space only when ${\displaystyle p=2.}$ If this identity is satisfied, the associated inner product is given by the polarization identity. In the case of real scalars, this gives:

For complex scalars, defining the inner product so as to be ${\displaystyle \mathbb {C} }$-linear in ${\displaystyle x,}$ antilinear in ${\displaystyle y,}$ the polarization identity gives:

To see that the parallelogram law is sufficient, one observes in the real case that ${\displaystyle \langle x,y\rangle }$ is symmetric, and in the complex case, that it satisfies the Hermitian symmetry property and ${\displaystyle \langle ix,y\rangle =i\langle x,y\rangle .}$ The parallelogram law implies that ${\displaystyle \langle x,y\rangle }$ is additive in ${\displaystyle x.}$ It follows that it is linear over the rationals, thus linear by continuity.

Several characterizations of spaces isomorphic (rather than isometric) to Hilbert spaces are available. The parallelogram law can be extended to more than two vectors, and weakened by the introduction of a two-sided inequality with a constant ${\displaystyle c\geq 1}$: Kwapień proved that if

for every integer ${\displaystyle n}$ and all families of vectors${\displaystyle \left\{x_{1},\ldots ,x_{n}\right\}\subseteq X,}$ then the Banach space ${\displaystyle X}$ is isomorphic to a Hilbert space.^[64] Here, ${\displaystyle \operatorname {Ave} _{\pm }}$ denotes the average over the ${\displaystyle 2^{n}}$ possible choices of signs ${\displaystyle \pm 1.}$ In the same article, Kwapień proved that the validity of a Banach-valued Parseval's theorem for the Fourier transform characterizes Banach spaces isomorphic to Hilbert spaces.

Lindenstrauss and Tzafriri proved that a Banach space in which every closed linear subspace is complemented (that is, is the range of a bounded linear projection) is isomorphic to a Hilbert space.^[65] The proof rests upon Dvoretzky's theorem about Euclidean sections of high-dimensional centrally symmetric convex bodies. In other words, Dvoretzky's theorem states that for every integer ${\displaystyle n,}$ any finite-dimensional normed space, with dimension sufficiently large compared to ${\displaystyle n,}$ contains subspaces nearly isometric to the ${\displaystyle n}$-dimensional Euclidean space.

The next result gives the solution of the so-called homogeneous space problem. An infinite-dimensional Banach space ${\displaystyle X}$ is said to be homogeneous if it is isomorphic to all its infinite-dimensional closed subspaces. A Banach space isomorphic to ${\displaystyle \ell ^{2}}$ is homogeneous, and Banach asked for the converse.^[66]

An infinite-dimensional Banach space is hereditarily indecomposable when no subspace of it can be isomorphic to the direct sum of two infinite-dimensional Banach spaces. The Gowers dichotomy theorem^[67] asserts that every infinite-dimensional Banach space ${\displaystyle X}$ contains, either a subspace ${\displaystyle Y}$ with unconditional basis, or a hereditarily indecomposable subspace ${\displaystyle Z,}$ and in particular, ${\displaystyle Z}$ is not isomorphic to its closed hyperplanes.^[68] If ${\displaystyle X}$ is homogeneous, it must therefore have an unconditional basis. It follows then from the partial solution obtained by Komorowski and Tomczak–Jaegermann, for spaces with an unconditional basis,^[69] that ${\displaystyle X}$ is isomorphic to ${\displaystyle \ell ^{2}.}$

Metric classification

If ${\displaystyle T:X\to Y}$ is an isometry from the Banach space ${\displaystyle X}$ onto the Banach space ${\displaystyle Y}$ (where both ${\displaystyle X}$ and ${\displaystyle Y}$ are vector spaces over ${\displaystyle \mathbb {R} }$), then the Mazur–Ulam theorem states that ${\displaystyle T}$ must be an affine transformation. In particular, if ${\displaystyle T(0_{X})=0_{Y},}$ this is ${\displaystyle T}$ maps the zero of ${\displaystyle X}$ to the zero of ${\displaystyle Y,}$ then ${\displaystyle T}$ must be linear. This result implies that the metric in Banach spaces, and more generally in normed spaces, completely captures their linear structure.

Topological classification

Finite dimensional Banach spaces are homeomorphic as topological spaces, if and only if they have the same dimension as real vector spaces.

Anderson–Kadec theorem (1965–66) proves^[70] that any two infinite-dimensional separable Banach spaces are homeomorphic as topological spaces. Kadec's theorem was extended by Torunczyk, who proved^[71] that any two Banach spaces are homeomorphic if and only if they have the same density character, the minimum cardinality of a dense subset.

Spaces of continuous functions

When two compact Hausdorff spaces ${\displaystyle K_{1}}$ and ${\displaystyle K_{2}}$ are homeomorphic, the Banach spaces ${\displaystyle C\left(K_{1}\right)}$ and ${\displaystyle C\left(K_{2}\right)}$ are isometric. Conversely, when ${\displaystyle K_{1}}$ is not homeomorphic to ${\displaystyle K_{2},}$ the (multiplicative) Banach–Mazur distance between ${\displaystyle C\left(K_{1}\right)}$ and ${\displaystyle C\left(K_{2}\right)}$ must be greater than or equal to ${\displaystyle 2,}$ see above the results by Amir and Cambern. Although uncountable compact metric spaces can have different homeomorphy types, one has the following result due to Milutin:^[72]

The situation is different for countably infinite compact Hausdorff spaces. Every countably infinite compact ${\displaystyle K}$ is homeomorphic to some closed interval of ordinal numbers

equipped with the order topology, where ${\displaystyle \alpha }$ is a countably infinite ordinal.^[74] The Banach space ${\displaystyle C(K)}$ is then isometric to C(⟨1, α⟩). When ${\displaystyle \alpha ,\beta }$ are two countably infinite ordinals, and assuming ${\displaystyle \alpha \leq \beta ,}$ the spaces C(⟨1, α⟩) and C(⟨1, β⟩) are isomorphic if and only if β < α^ω.^[75] For example, the Banach spaces are mutually non-isomorphic.

Examples

Glossary of symbols for the table below:

• ${\displaystyle \mathbb {F} }$ denotes the field of real numbers ${\displaystyle \mathbb {R} }$ or complex numbers ${\displaystyle \mathbb {C} .}$
• ${\displaystyle K}$ is a compact Hausdorff space.
• ${\displaystyle p,q\in \mathbb {R} }$ are real numbers with ${\displaystyle 1<p,q<\infty }$ that are Hölder conjugates, meaning that they satisfy ${\displaystyle {\frac {1}{q}}+{\frac {1}{p}}=1}$ and thus also ${\displaystyle q={\frac {p}{p-1}}.}$
• ${\displaystyle \Sigma }$ is a ${\displaystyle \sigma }$-algebra of sets.
• ${\displaystyle \Xi }$ is an algebra of sets (for spaces only requiring finite additivity, such as the ba space).
• ${\displaystyle \mu }$ is a measure with variation ${\displaystyle \mu .}$ A positive measure is a real-valued positive set function defined on a ${\displaystyle \sigma }$-algebra which is countably additive.

Classical Banach spaces
	Dual space	Reflexive	weakly sequentially complete	Norm		Notes
${\displaystyle \mathbb {F} ^{n}}$	${\displaystyle \mathbb {F} ^{n}}$	Yes	Yes	${\displaystyle \ x\ _{2}}$	${\displaystyle =\left(\sum _{i=1}^{n} x_{i} ^{2}\right)^{1/2}}$	Euclidean space
${\displaystyle \ell _{p}^{n}}$	${\displaystyle \ell _{q}^{n}}$	Yes	Yes	${\displaystyle \ x\ _{p}}$	${\displaystyle =\left(\sum _{i=1}^{n} x_{i} ^{p}\right)^{\frac {1}{p}}}$
${\displaystyle \ell _{\infty }^{n}}$	${\displaystyle \ell _{1}^{n}}$	Yes	Yes	${\displaystyle \ x\ _{\infty }}$	${\displaystyle =\max \nolimits _{1\leq i\leq n} x_{i} }$
${\displaystyle \ell ^{p}}$	${\displaystyle \ell ^{q}}$	Yes	Yes	${\displaystyle \ x\ _{p}}$	${\displaystyle =\left(\sum _{i=1}^{\infty } x_{i} ^{p}\right)^{\frac {1}{p}}}$
${\displaystyle \ell ^{1}}$	${\displaystyle \ell ^{\infty }}$	No	Yes	${\displaystyle \ x\ _{1}}$	${\displaystyle =\sum _{i=1}^{\infty }\left x_{i}\right }$
${\displaystyle \ell ^{\infty }}$	${\displaystyle \operatorname {ba} }$	No	No	${\displaystyle \ x\ _{\infty }}$	${\displaystyle =\sup \nolimits _{i}\left x_{i}\right }$
${\displaystyle \operatorname {c} }$	${\displaystyle \ell ^{1}}$	No	No	${\displaystyle \ x\ _{\infty }}$	${\displaystyle =\sup \nolimits _{i}\left x_{i}\right }$
${\displaystyle c_{0}}$	${\displaystyle \ell ^{1}}$	No	No	${\displaystyle \ x\ _{\infty }}$	${\displaystyle =\sup \nolimits _{i}\left x_{i}\right }$	Isomorphic but not isometric to ${\displaystyle c.}$
${\displaystyle \operatorname {bv} }$	${\displaystyle \ell ^{\infty }}$	No	Yes	${\displaystyle \ x\ _{bv}}$	${\displaystyle =\left x_{1}\right +\sum _{i=1}^{\infty }\left x_{i+1}-x_{i}\right }$	Isometrically isomorphic to ${\displaystyle \ell ^{1}.}$
${\displaystyle \operatorname {bv} _{0}}$	${\displaystyle \ell ^{\infty }}$	No	Yes	${\displaystyle \ x\ _{bv_{0}}}$	${\displaystyle =\sum _{i=1}^{\infty }\left x_{i+1}-x_{i}\right }$	Isometrically isomorphic to ${\displaystyle \ell ^{1}.}$
${\displaystyle \operatorname {bs} }$	${\displaystyle \operatorname {ba} }$	No	No	${\displaystyle \ x\ _{bs}}$	${\displaystyle =\sup \nolimits _{n}\left \sum _{i=1}^{n}x_{i}\right }$	Isometrically isomorphic to ${\displaystyle \ell ^{\infty }.}$
${\displaystyle \operatorname {cs} }$	${\displaystyle \ell ^{1}}$	No	No	${\displaystyle \ x\ _{bs}}$	${\displaystyle =\sup \nolimits _{n}\left \sum _{i=1}^{n}x_{i}\right }$	Isometrically isomorphic to ${\displaystyle c.}$
${\displaystyle B(K,\Xi )}$	${\displaystyle \operatorname {ba} (\Xi )}$	No	No	${\displaystyle \ f\ _{B}}$	${\displaystyle =\sup \nolimits _{k\in K} f(k) }$
${\displaystyle C(K)}$	${\displaystyle \operatorname {rca} (K)}$	No	No	${\displaystyle \ x\ _{C(K)}}$	${\displaystyle =\max \nolimits _{k\in K} f(k) }$
${\displaystyle \operatorname {ba} (\Xi )}$	?	No	Yes	${\displaystyle \ \mu \ _{ba}}$	${\displaystyle =\sup \nolimits _{S\in \Sigma } \mu (S)}$
${\displaystyle \operatorname {ca} (\Sigma )}$	?	No	Yes	${\displaystyle \ \mu \ _{ba}}$	${\displaystyle =\sup \nolimits _{S\in \Sigma } \mu (S)}$	A closed subspace of ${\displaystyle \operatorname {ba} (\Sigma ).}$
${\displaystyle \operatorname {rca} (\Sigma )}$	?	No	Yes	${\displaystyle \ \mu \ _{ba}}$	${\displaystyle =\sup \nolimits _{S\in \Sigma } \mu (S)}$	A closed subspace of ${\displaystyle \operatorname {ca} (\Sigma ).}$
${\displaystyle L^{p}(\mu )}$	${\displaystyle L^{q}(\mu )}$	Yes	Yes	${\displaystyle \ f\ _{p}}$	${\displaystyle =\left(\int f ^{p}\,d\mu \right)^{\frac {1}{p}}}$
${\displaystyle L^{1}(\mu )}$	${\displaystyle L^{\infty }(\mu )}$	No	Yes	${\displaystyle \ f\ _{1}}$	${\displaystyle =\int f \,d\mu }$	The dual is ${\displaystyle L^{\infty }(\mu )}$ if ${\displaystyle \mu }$ is ${\displaystyle \sigma }$-finite.
${\displaystyle \operatorname {BV} ([a,b])}$	?	No	Yes	${\displaystyle \ f\ _{BV}}$	${\displaystyle =V_{f}([a,b])+\lim \nolimits _{x\to a^{+}}f(x)}$	${\displaystyle V_{f}([a,b]).}$ is the total variation of ${\displaystyle f}$
${\displaystyle \operatorname {NBV} ([a,b])}$	?	No	Yes	${\displaystyle \ f\ _{BV}}$	${\displaystyle =V_{f}([a,b])}$	${\displaystyle \operatorname {NBV} ([a,b])}$ consists of ${\displaystyle \operatorname {BV} ([a,b])}$ functions such that ${\displaystyle \lim \nolimits _{x\to a^{+}}f(x)=0}$
${\displaystyle \operatorname {AC} ([a,b])}$	${\displaystyle \mathbb {F} +L^{\infty }([a,b])}$	No	Yes	${\displaystyle \ f\ _{BV}}$	${\displaystyle =V_{f}([a,b])+\lim \nolimits _{x\to a^{+}}f(x)}$	Isomorphic to the Sobolev space ${\displaystyle W^{1,1}([a,b]).}$
${\displaystyle C^{n}([a,b])}$	${\displaystyle \operatorname {rca} ([a,b])}$	No	No	${\displaystyle \ f\ }$	${\displaystyle =\sum _{i=0}^{n}\sup \nolimits _{x\in [a,b]}\left f^{(i)}(x)\right }$	Isomorphic to ${\displaystyle \mathbb {R} ^{n}\oplus C([a,b]),}$ essentially by Taylor's theorem.

Derivatives

Several concepts of a derivative may be defined on a Banach space. See the articles on the Fréchet derivative and the Gateaux derivative for details. The Fréchet derivative allows for an extension of the concept of a total derivative to Banach spaces. The Gateaux derivative allows for an extension of a directional derivative to locally convex topological vector spaces. Fréchet differentiability is a stronger condition than Gateaux differentiability. The quasi-derivative is another generalization of directional derivative that implies a stronger condition than Gateaux differentiability, but a weaker condition than Fréchet differentiability.

Generalizations

Several important spaces in functional analysis, for instance the space of all infinitely often differentiable functions ${\displaystyle \mathbb {R} \to \mathbb {R} ,}$ or the space of all distributions on ${\displaystyle \mathbb {R} ,}$ are complete but are not normed vector spaces and hence not Banach spaces. In Fréchet spaces one still has a complete metric, while LF-spaces are complete uniform vector spaces arising as limits of Fréchet spaces.

See also

• Space (mathematics) – Mathematical set with some added structure
  • Fréchet space – A locally convex topological vector space that is also a complete metric space
  • Hardy space – Concept within complex analysis
  • Hilbert space – Generalization of Euclidean space allowing infinite dimensions
  • L-semi-inner product – Generalization of inner products that applies to all normed spaces
  • ${\displaystyle L^{p}}$ space – Function spaces generalizing finite-dimensional p norm spaces
  • Sobolev space – Vector space of functions in mathematics
  • Banach lattice
• Banach manifold – Manifold modeled on Banach spaces
  • Banach bundle
• Distortion problem
• Interpolation space
• Locally convex topological vector space – A vector space with a topology defined by convex open sets
• Smith space
• Topological vector space – Vector space with a notion of nearness

Notes

References

Bibliography

• Bachman, George; Narici, Lawrence (2000). Functional Analysis (Second ed.). Mineola, New York: Dover Publications. ISBN 978-0486402512. OCLC 829157984.
• Banach, Stefan (1932). Théorie des Opérations Linéaires [Theory of Linear Operations] (PDF). Monografie Matematyczne (in French). Vol. 1. Warszawa: Subwencji Funduszu Kultury Narodowej. Zbl 0005.20901. Archived from the original (PDF) on 2014-01-11. Retrieved 2020-07-11.
• Beauzamy, Bernard (1985) [1982], Introduction to Banach Spaces and their Geometry (Second revised ed.), North-Holland.* Bourbaki, Nicolas (1987) [1981]. Topological Vector Spaces: Chapters 1–5. Éléments de mathématique. Translated by Eggleston, H.G.; Madan, S. Berlin New York: Springer-Verlag. ISBN 3-540-13627-4. OCLC 17499190.
• Bessaga, C.; Pełczyński, A. (1975), Selected Topics in Infinite-Dimensional Topology, Monografie Matematyczne, Warszawa: Panstwowe wyd. naukowe.
• Carothers, Neal L. (2005), A short course on Banach space theory, London Mathematical Society Student Texts, vol. 64, Cambridge: Cambridge University Press, pp. xii+184, ISBN 0-521-84283-2.
• Conway, John (1990). A course in functional analysis. Graduate Texts in Mathematics. Vol. 96 (2nd ed.). New York: Springer-Verlag. ISBN 978-0-387-97245-9. OCLC 21195908.
• Diestel, Joseph (1984), Sequences and series in Banach spaces, Graduate Texts in Mathematics, vol. 92, New York: Springer-Verlag, pp. xii+261, ISBN 0-387-90859-5.
• Dunford, Nelson; Schwartz, Jacob T. with the assistance of W. G. Bade and R. G. Bartle (1958), Linear Operators. I. General Theory, Pure and Applied Mathematics, vol. 7, New York: Interscience Publishers, Inc., MR 0117523
• Edwards, Robert E. (1995). Functional Analysis: Theory and Applications. New York: Dover Publications. ISBN 978-0-486-68143-6. OCLC 30593138.
• Grothendieck, Alexander (1973). Topological Vector Spaces. Translated by Chaljub, Orlando. New York: Gordon and Breach Science Publishers. ISBN 978-0-677-30020-7. OCLC 886098.
• Khaleelulla, S. M. (1982). Counterexamples in Topological Vector Spaces. Lecture Notes in Mathematics. Vol. 936. Berlin, Heidelberg, New York: Springer-Verlag. ISBN 978-3-540-11565-6. OCLC 8588370.
• Lindenstrauss, Joram; Tzafriri, Lior (1977), Classical Banach Spaces I, Sequence Spaces, Ergebnisse der Mathematik und ihrer Grenzgebiete, vol. 92, Berlin: Springer-Verlag, ISBN 3-540-08072-4.
• Megginson, Robert E. (1998), An introduction to Banach space theory, Graduate Texts in Mathematics, vol. 183, New York: Springer-Verlag, pp. xx+596, ISBN 0-387-98431-3.
• Narici, Lawrence; Beckenstein, Edward (2011). Topological Vector Spaces. Pure and applied mathematics (Second ed.). Boca Raton, FL: CRC Press. ISBN 978-1584888666. OCLC 144216834.
• Robertson, Alex P.; Robertson, Wendy J. (1980). Topological Vector Spaces. Cambridge Tracts in Mathematics. Vol. 53. Cambridge England: Cambridge University Press. ISBN 978-0-521-29882-7. OCLC 589250.
• Rudin, Walter (1991). Functional Analysis. International Series in Pure and Applied Mathematics. Vol. 8 (Second ed.). New York, NY: McGraw-Hill Science/Engineering/Math. ISBN 978-0-07-054236-5. OCLC 21163277.
• Ryan, Raymond A. (2002), Introduction to Tensor Products of Banach Spaces, Springer Monographs in Mathematics, London: Springer-Verlag, pp. xiv+225, ISBN 1-85233-437-1.
• Schaefer, Helmut H.; Wolff, Manfred P. (1999). Topological Vector Spaces. GTM. Vol. 8 (Second ed.). New York, NY: Springer New York Imprint Springer. ISBN 978-1-4612-7155-0. OCLC 840278135.
• Swartz, Charles (1992). An introduction to Functional Analysis. New York: M. Dekker. ISBN 978-0-8247-8643-4. OCLC 24909067.
• Trèves, François (2006) [1967]. Topological Vector Spaces, Distributions and Kernels. Mineola, N.Y.: Dover Publications. ISBN 978-0-486-45352-1. OCLC 853623322.
• Wilansky, Albert (2013). Modern Methods in Topological Vector Spaces. Mineola, New York: Dover Publications, Inc. ISBN 978-0-486-49353-4. OCLC 849801114.
• Wojtaszczyk, Przemysław (1991), Banach spaces for analysts, Cambridge Studies in Advanced Mathematics, vol. 25, Cambridge: Cambridge University Press, pp. xiv+382, ISBN 0-521-35618-0.

External links

• "Banach space", Encyclopedia of Mathematics, EMS Press, 2001 [1994]
• Weisstein, Eric W. "Banach Space". MathWorld.