삼각형의 세 면 모두에 접하는 원
A 근친상간인센티브 가 있는 삼각형(I {\displaystyle I}), JA displaystyle {A JB {\ displaysty J_ {C}), 내부 각도 이등분자 및 외부 각도 이등분자 녹색 기하학 에서 삼각형 의 근골 이나 새겨진 원은 삼각형에 포함된 가장 큰 원 이다. 삼각형은 삼면에 접한다 . 근친상간 중심은 삼각형의 인센티브 로 불리는 삼각형의 중심 이다.[1]
삼각형의 외부 또는 생략된 원은 [2] 다른 두 개의 연장선 에 접한다. 모든 삼각형에는 세 개의 뚜렷한 외관이 있는데, 각각은 삼각형의 한쪽 면에 접한다.[3]
인센티브 내부 각도 이등분자 가 교차하는 것으로서 찾을 수 있다.[3] [4] excircle의 중심은 한 각(예를 들어 꼭지점 {\displaystyle }) 외부 이등분자의 교차점이다. 이 방접원. 중심은 excenter 꼭지점에 비해 한{A\displaystyle}또는 A{A\displaystyle}.[3]의 excenter기 때문에 각도의 내부 이등분선은 외부 이등분선에 수직이다라고 불린 것은 내접원의 3방접원. 센터의 중심으로 같이orthocentric 시스템을 형성한다..[5]:우편 182
모든 일반 폴리곤 은 모든 면에 접하는 경계가 있지만 모든 폴리곤이 접하는 것은 아니다; 접선 폴리곤 은 접선이다. 원의 접선도 참조 하십시오.
근친상간 및 인센티브 제공자 Suppose △ A B C {\displaystyle \triangle ABC} r {\displaystyle r} I {\displaystyle I} a {\displaystyle a} B C {\displaystyle BC} b {\displaystyle b} A C {\displaystyle AC} c {\displaystyle c} A B {\displaystyle AB} T A {\displaystyle T_{A}} T B {\displaystyle T_{B}} T C {\displaystyle T_{C}} B C {\displaystyle BC} A C {\displaystyle AC} A B {\displaystyle AB}
장려자 인센티브는 ∠ A B C , ∠ C A ∠ A C 각도 이등분자 ( ABC,\angle BCA,{\text, }}\angle BAC}
꼭지점 A {\displaystyle A} 인센티브 {\displaystyle I} [citation needed
d ( A , I ) = c 죄를 짓다 ( B 2 ) cas ( C 2 ) = b 죄를 짓다 ( C 2 ) cas ( B 2 ) . {\displaystyle d(A,I)=c{\frac {\sin \왼쪽({\frac {B}{2}}\오른쪽) }}{\cos \left({\frac {C}{2}}\오른쪽)}}}=b{\frac {\sin \left({\frac {C}{2}}\오른쪽) }}{\cos \left({\frac {B}{2}}\오른쪽)}}}. } 삼선 좌표 삼각형의 한 점에 대한 삼선 좌표 는 삼각형 변에 대한 모든 거리의 비율이다. 인센티브 제공자는 삼각형의 모든 면으로부터 동일한 거리이기 때문에, 인센티브 제공자의 3행 좌표는 다음과[6]
1 : 1 : 1. 1:1:1. } 편심 좌표 삼각형 점의 이심 좌표 는 점이 삼각형 꼭지점 위치의 가중 평균이 되도록 가중치를 부여한다. 인센티브를 위한 2차 좌표는 다음과[citation needed 같다.
a : b : c {\displaystyle \ a:b:c} 여기 displaystyle a b {\displaystyle b} {\displaystyle c} sine 의 법칙을 사용하여) 다음에 의해 동등하게 (sine의 법칙을 사용하여) 된다.
죄를 짓다 ( A ) : 죄를 짓다 ( B ) : 죄를 짓다 ( C ) \displaystyle \sin(A):\sin(B):\sin(C)} 여기서 A {\displaystyle A B {\displaystyle B} C {\displaystyle C}
데카르트 좌표, 평행 좌표. 인센티브자의 데카르트 좌표 는 둘레에 상대적인 삼각형의 측면 길이(즉, 위에 제시된 이심 좌표를 사용하여 통일성에 합치도록 정규화)를 가중치로 사용하여 정점 세 개의 좌표에 대한 가중 평균이다. 가중치는 양성이므로 인센티브는 위에서 설명한 삼각형 안에 위치한다. If the three vertices are located at ( x a , y a ) {\displaystyle (x_{a},y_{a})} ( x b , y b ) {\displaystyle (x_{b},y_{b})} ( x c , y c ) {\displaystyle (x_{c},y_{c})} a {\displaystyle a} b {\displaystyle b} d [\displaystyle }, [citation needed
( a x a + b x b + c x c a + b + c , a y a + b y b + c y c a + b + c ) = a ( x a , y a ) + b ( x b , y b ) + c ( x c , y c ) a + b + c . {\displaystyle \left({\frac {ax_{a}+bx_{b}+cx_{c}}{a+b+c}},{\frac {ay_{a}+by_{b}+cy_{c}}{a+b+c}}\right)={\frac {a\left(x_{a},y_{a}\right)+b\left(x_{b},y_{b}\right)+c\left(x_{c},y_{c}\right)}{a+b+c}}. } 반지름 길이 {\displaystyle a {\displaystyle b c {\displaystyle c} r {\displaystyle r} [7]
r s a s s s {\displaystyle ={\sqrt {\frac {(s-a)(s-b c)}}{s}}}}, s b c {\displaystystyle =(a+c)/ 2). } 헤론의 공식 을 봐
정점까지의 거리 " A \triangle ABC} 인센티브 displaystyle I} , [8]
I A ⋅ I A C A ⋅ A B + I B ⋅ I B A B ⋅ B C + I C ⋅ I C B C ⋅ C A = 1. 디스플레이 스타일(displaystyle {\frac} IA\cdot IA}{ CA\cdot AB}+{\frac {IB\cdot IB}{ AB\cdot BC}}+{\frac {IC\cdot IC}{BC\cdot CA}=1.} 게다가[9]
I A ⋅ I B ⋅ I C = 4 R r 2 , {\displaystyle IA\cdot IB\cdot IC=4Rr^{2},} 여기서 R {\displaystyle R} r {\displaystyle r} 원곡선 및 인반선 이다.
기타 속성 삼각형 중심들의 집합은 좌표-현상 좌표의 곱셈에 따라 집단 의 구조를 부여받을 수 있다. 이 집단에서, 인센티브는 정체성 요소 를 형성한다.[6]
근친 및 반지름 특성 정점과 가장 가까운 접점 사이의 거리 정점에서 가장 가까운 두 지점까지의 거리는 동일하다. 예를 들어,[10]
d ( A , T B ) = d ( A , T C ) = 1 2 ( b + c − a ) . {\displaystyle d\ft(A,T_{B}\right)=d\left(A,T_{C}\right)={\frac {1}{1}:{2}}(b+c-a). } 기타 속성 근방의 접선점을 x {\displaystyle x} {\displaystyle }, y {\displaystyle y} {\displaystyle z z {\displaystyle z} x {\displaystystyle } . 그러면 근친은 반경을[11]
r = x y z x + y + z {\displaystyle r={\sqrt {\frac {xyz}{x+y+z} }}} 그리고 삼각형의 넓이는
Δ = x y z ( x + y + z ) . {\displaystyle \Delta ={\sqrt {xyz(x+y+z) }}.} 길이 측면 a {\displaystyle a }, {\displaystyle }, c {\ displaystyle h_ {b h {\ displaystyle h_{c r {\displaystystyle r} 이러한 조화 평균 의 1/3이다 es; 즉,[12]
r = 1 1 h a + 1 h b + 1 h c . {\displaystyle r={\frac {1}{\frac {1}{h_{a}}}+{\frac {1}{h_{b}}+{\frac {1}{c}}}}. } {\displaystyle a b {\displaystyle b} c {\displaystyle c} 변이 r {\displaystyle r} 원주근 반지름 R {\\displaystyle R} [5] : 189, #298(d)
r R = a b c 2 ( a + b + c ) . {\displaystyle rR={\frac {abc}{2(a+b+c) }}.} 측면, 근교 반지름 및 원주 반지름 사이의 일부 관계는 다음과 같다.[13]
a b + b c + c a = s 2 + ( 4 R + r ) r , a 2 + b 2 + c 2 = 2 s 2 − 2 ( 4 R + r ) r . {\displaystyle {\begin}ab+bc+ca&=s^{2}+(4R+r)r,\a^{2}+b^{2}+c^{2} }}&=2s^{2}-2(4R+r)r. \end{정렬}}} 삼각형의 영역과 둘레를 반으로 갈라놓는 삼각형을 통과하는 선은 삼각형의 인센티브자(근골의 중심)를 통과한다. 주어진 삼각형에는 이것들 중 하나, 둘 또는 셋이 있다.[14]
A displaystyle \triangle ABC} 중심부 {\displaystyle I} [15] ,
I A ⋅ I A C A ⋅ A B + I B ⋅ I B A B ⋅ B C + I C ⋅ I C B C ⋅ C A = 1 디스플레이 스타일(displaystyle {\frac} IA\cdot IA}{ CA\cdot AB}+{\frac {IB\cdot IB}{ AB\cdot BC}}+{\frac {IC\cdot IC}{BC\cdot CA}=1} 그리고[16] : 121, #84
I A ⋅ I B ⋅ I C = 4 R r 2 . {\displaystyle IA\cdot IB\cdot IC=4Rr^{2}. } 근친 반경은 고도 합계의 9분의 1 이하가 아니다.[17] : 289
인센티브자 I {\displaystyle I} O {\displaystyle O} [18] : 232
O I 2 R 2 r {\displaystyle OI^{2}=R(R-2r 인센티브자에서 9점 원 의 중심 {\displaystyle } [18] : 232
I N = 1 2 ( R − 2 r ) < 1 2 R . {\displaystyle IN={\frac {1}{1}{1}{2}}(R-2r)<{\frac {1}{2}}R. } 인센티브는 내측 삼각형 (정점이 측면의 중간점인 경우)에 있다.[18] : 233, Lemma 1
삼각형 영역과의 관계 근친의 반경은 삼각형의 면적 과 관련이 있다.[19] 삼각형 면적에 대한 근골 면적 비율은 π 3 3 displaystyle {\tfrac {\pi }{3{\sqrt{ [20] .
Suppose △ A B C {\displaystyle \triangle ABC} r {\displaystyle r} I {\displaystyle I} a {\displaystyle a} B C {\displaystyle BC} b {\displaystyle b} A C {\displaystyle AC} c {\displaystyle c} A B {\displaystyle AB T C {\ displaystyle T_ C} A B {\displaystyle AB} ∠ A T C I {\displaystyle \angle AT_{C}I } 따라서 T C {\displaystyle T_{C} I} altitude of △ I A B {\displaystyle \triangle IAB} △ I A B {\displaystyle \triangle IAB} c {\displaystyle c} r {\displaystyle r} 1 2 c r {\displaystyle {\tfrac {1}{2}}cr} △ I A C {\displaystyle \triangle IAC} area 1 2 b r {\displaystyle {\tfrac {1}{2}}br} △ I B C {\displaystyle \triangle IBC} 1 2 a r {\displaystyle {\tfrac {1}{2}}ar} △ A B C {\displaystyle \triangle ABC} Δ of △ A B C {\displaystyle \Delta {\text{ of }}\trian gle ABC} [citation needed 는) 다음과 같다.
Δ 2 a b c r s r , displaystyle \Delta ={\frac{1}{ 1}{{\ a+b+c)=r,}, r Δs {\displaysty ={\fraca},},},},},} 여기서 Δ {\displaystyle \Delta } ( A C {\displaystyle \triangle ABC} s 1 (+ b ) {\displaysty s={\tfrac{1 1}:{ a+b+c)} 반퍼미터 다
다른 공식의 경우 I displaystyle \triangle IT_{C} . 한 {\displaystyle r } r cot cot ){\ displaystysty \cot \left({\frac {A}{2}}\오른쪽 I B ′ A {\displaystyle \triangle IB'A} . [citation needed
Δ = r 2 ( 요람을 달다 ( A 2 ) + 요람을 달다 ( B 2 ) + 요람을 달다 ( C 2 ) ) . {\displaystyle \Delta =r^{2}\왼쪽(\cot \왼쪽({\frac {A}{2}}\오른쪽)+\cot \왼쪽({\frac {B}{2}}\오른쪽)+\cot \왼쪽({\frac {C}{2}}\오른쪽)\오른쪽). } 게르곤 삼각형과 점 삼각형, B {\displaystyle \triangle ABC I {\displaystyle I} (T A B C {\ displaystyle \triangle T_{A}T_{B}) T_{C G e {\ displaystyle G_{e 게르고네 삼각형 (A B C {\displaystyle \triangle ABC A {\displaystyle A} A {\ displaystyle T_{A} .
이 , T B T C {\ displaystyle \triangle T_{A}T_{B} T_{C 접촉 삼각형 인터치 삼각형이라고 도 하며, A {\displaystyle \triangle ABC}
K T = K 2 r 2 s a b c {\displaystyle K_{ T}=K{\frac {2r^{2}s}{abc}}}} 여기서 K {\displaystyle K r {\displaystyle r} {\displaystyle s} 반지름 및 반시미터이며, {\displaystyle a b {\ displaystytle b c} 길이 이것은 바깥쪽 삼각형 의 그것과 같은 영역이다.[21]
A A displaystyle AT_{A B T {\ displaystyle BT_{B}, T {\ displaystyle CT_{C} Gergonne 지점 이라는 단일 지점에서 교차하며e {\ displaysty e}( 중심 X)표시 된다7 . 게르곤느 지점은 자신의 중앙에 뚫린 열린 직교좌현 원반 안에 있으며, 그 안에서 어떤 지점도 될 수 있다.[22]
삼각형의 게르곤느 점에는 게르곤느 삼각형의 시메디언 점이라는 점 등 여러 가지 특성이 있다.[23]
인터치 삼각형의 정점에 대한 트리린 좌표 는 다음과[citation needed 같다.
꼭지점 T A = 0 : 초 2 ( B 2 ) : 초 2 ( C 2 ) {\displaystyle {\text{vertex}\,T_{ A}=0:\sec ^{2}\왼쪽({\frac {B}{2}}\\오른쪽): \sec ^{2}\왼쪽({\frac {C}{2}}\오른쪽)} 꼭지점 T B = 초 2 ( A 2 ) : 0 : 초 2 ( C 2 ) {\displaystyle {\text{vertex}}\,T_{B}=\sec ^{2}\왼쪽({\frac {A}{2}}\오른쪽):0:\sec ^{2}\왼쪽({\frac {C}{2}}\오른쪽)}}} 꼭지점 T C = 초 2 ( A 2 ) : 초 2 ( B 2 ) : 0. {\displaystyle {\text{vertex}\,T_{C}=\sec ^{2}\왼쪽({\frac {A}{2}}\오른쪽): \sec ^{2}\왼쪽({\frac {B}{2}}\오른쪽):0. } 게르곤 포인트에 대한 트리린 좌표는 다음과[citation needed 같다.
초 2 ( A 2 ) : 초 2 ( B 2 ) : 초 2 ( C 2 ) , {\displaystyle \sec ^{2}\왼쪽({\frac {A}{2}}\오른쪽): \sec ^{2}\왼쪽({\frac {B}{2}}\오른쪽): \sec ^{2}\왼쪽({\frac {C}{2}}\오른쪽),} 또는 동등하게, 시네스의 법칙 에 따라
b c b + c − a : c a c + a − b : a b a + b − c . {\displaystyle {\frac {b+c-a}, {c+a}, {c+a-a}, {c+a-b}, {a+b}, {a+b-c}. } 엑시클과 엑시터 근친I {\displaystyle I}), J A {\ displaystyle J_{A J {\ displaystyle J_{B C {\ displaysty J_{C}), 각도 이등분자 와 외부 각도 녹색 삼각형의 외부 또는 생략된 원은 [24] 다른 두 개의 연장선 에 접한다. 모든 삼각형에는 세 개의 뚜렷한 외관이 있는데, 각각은 삼각형의 한쪽 면에 접한다.[3]
excircle의 중심은 한 각(예를 들어 꼭지점 {\displaystyle }) 외부 이등분자의 교차점이다. 이 방접원. 중심은 excenter 꼭지점에 비해 한{A\displaystyle}또는 A{A\displaystyle}.[3]의 excenter기 때문에 각도의 내부 이등분선은 외부 이등분선에 수직이다라고 불린 것은 내접원의 3방접원. 센터의 중심으로 같이orthocentric 시스템을 형성한다..[5]:182
외향 좌표 While the incenter of △ A B C {\displaystyle \triangle ABC} trilinear coordinates 1 : 1 : 1 {\displaystyle 1:1:1} − 1 : 1 : 1 {\displaystyle -1:1:1} 1 : − 1 : 1 {\displaystyle 1:-1:1} 1 : 1 : − 1 {\displaystyle 1:1:-1} [citation needed
엑라디야속 엑스트라디악의 반지름을 엑스트라디악 이라고 한다.
A {\displaystyle }( JA {\ displaystyle J_{A} B {\displaystyle BC [25] [26]
r a = r s s − a = s ( s − b ) ( s − c ) s − a , {\displaystyle r_{a}={\frac {rs}{s-a}}={\sqrt {\frac {s(s-b)(s-c)}{s-a}}},} s = 1 2 ( a + b + c ) . {\displaystyle s={\tfrac {1}{2}}(a+b+c). } 헤론의 공식 을 봐
exradii 공식의[27] 기타 속성 위의 공식으로 볼 때, 근골은 항상 근골보다 크고, 가장 큰 근골은 가장 긴 면에 접하는 것이고, 가장 작은 근골은 가장 짧은 면에 접하는 것임을 알 수 있다. 또한 이러한 공식을 결합하면 다음과 같은 결과가 나온다.[28]
Δ = r r a r b r c . {\displaystyle \Delta ={\sqrt {rr_{a}r_{b}r_{c}}}. } 기타 excircle 특성 신체의 원형 선체 는 내적으로 각 신체에 접하고 있으며 따라서 아폴로니우스 원 이다.[29] 이 아폴로니우스 원의 반지름은 r 2 2 4 r {\ displaystyle {\tfrac {r^{2}+s^{2}}:{4r}}}} 여기 {\displaystyle r} 반지름 displaysty [30]
다음 관계는 inradius r {\displaystyle }, {\displaystyle R }, 세미퍼미터 {\displaystyle }, r displaysty r_{a }, r {\ displaystysty r_ { c c} . [13]
r a + r b + r c = 4 R + r , r a r b + r b r c + r c r a = s 2 , r a 2 + r b 2 + r c 2 = ( 4 R + r ) 2 − 2 s 2 . {\displaystyle {\begin{aligned}r_{a}+r_{b}+r_{c}&=4R+r,\\r_{a}r_{b}+r_{b}r_{c}+r_{c}r_{a}&=s^{2},\\r_{a}^{2}+r_{b}^{2}+r_{c}^{2}&=\left(4R+r\right)^{2}-2s^{2}. \end{정렬}}} 세 개의 운동 중심부를 통과하는 원은 반지름 2 R [\displaystyle 2R} . [13]
H {\displaystyle H} ( B C {\displaystyle \triangle ABC} [13] 직교점 이라면,
r a + r b + r c + r = A H + B H + C H + 2 R , r a 2 + r b 2 + r c 2 + r 2 = A H 2 + B H 2 + C H 2 + ( 2 R ) 2 . {\displaystyle {\displaysty}r_{a}+r_{b}+r_{c}+r&= AH+BH+CH+2R,\r_{a}^{2}+r_{b}^{2}+r_{c}^{2}+r^{2}+r^{2}+r^{2}&= AH^{2}+B H^{2}+CH^{2}+(2R)^{2}. \end{정렬}}} 나글 삼각형과 나글 포인트 익스터치(- T A B T {\ displaystyle \triangle T_{A}T_{B}) T_{C A B C {\displaystyle \ triangle ABC}) Nagel 지점 (N {\displaystyle N 주황색 원은 삼각형의 원 이다. The Nagel triangle or extouch triangle of △ A B C {\displaystyle \triangle ABC} T A {\displaystyle T_{A}} T B {\displaystyle T_{B}} T C {\displaystyle T_{C}} △ A B C {\displaystyle \triangle ABC} 여기서 T A {\ displaystyle T_{A} A {\displaystyle A} . 이것=T A T B T C {\ displaystyle \triangle T_{A}T_{B} T_{C}} A C {\displaystyle \triangle ABC} extouch 삼각형이라고 도 한다. 원곡선 , T T B C {\ displaystyle \triangle T_{A}T_{B}" T_{C}} 만다트 원 이라고 한다.[citation needed
A T A {\ displaystyle AT_{A B T B {\ displaystyle BT_{B}, C C {\ displaystyle CT_{C} 분할 한다.[citation needed
A B + B T A = A C + C T A = 1 2 ( A B + B C + A C ) . (\displaystyle AB+) BT_{A}=AC+ CT_{A}={\frac {1}{1}:{2}}\왼쪽(AB+) BC+AC\right). } 분할자는 단일 점, 즉 삼각형의 Nagel 점 N a displaystyle {a}}( 삼각형 중심 8 X)에서 교차한다.
바깥쪽 삼각형의 정점에 대한 삼각형 좌표는 다음과[citation needed 같이 주어진다.
꼭지점 T A = 0 : csc 2 ( B 2 ) : csc 2 ( C 2 ) {\displaystyle {\text{vertex}\,T_{ A}=0:\csc ^{2}\왼쪽({\frac {B}{2}}\오른쪽):\csc ^{2}\왼쪽({\frac {C}{2}}\오른쪽)}} 꼭지점 T B = csc 2 ( A 2 ) : 0 : csc 2 ( C 2 ) {\displaystyle {\text{vertex}\,T_{B}=\csc ^{2}\왼쪽({\frac {A}{2}}\오른쪽):0:\csc ^{2}\왼쪽({\frac {C}{2}}\오른쪽)}}} 꼭지점 T C = csc 2 ( A 2 ) : csc 2 ( B 2 ) : 0. {\displaystyle {\text{vertex}}\,T_{C}=\csc ^{2}\좌측({\frac {A}{2}}\우측):\csc ^{2}\좌측({\frac {B}{2}}\우측):0. } Nagel 지점의 트리린 좌표는 다음과[citation needed 같다.
csc 2 ( A 2 ) : csc 2 ( B 2 ) : csc 2 ( C 2 ) , {\displaystyle \csc^{2}\왼쪽({\frac {A}{2}}\오른쪽):\csc ^{2}\왼쪽({\frac {B}{2}}\오른쪽)\csc ^{2}\왼쪽({\frac {C}{2}}\오른쪽),}} 또는 동등하게, 시네스의 법칙 에 따라
b + c − a a : c + a − b b : a + b − c c . {\displaystyle {\frac {b+c-a}{a}}}Mos\\frac {c+a-b}{b}}Mos}Ms\frac {a+b-c}{c}}}. } 나겔 점은 게르곤느 점의 동위원소 결합점 이다.[citation needed
관련 구성 9점 원 및 Feuerbach 점 기하학 에서 9점 원은 주어진 삼각형 에 대해 구성할 수 있는 원 이다. 그것은 삼각형에서 정의된 9개의 유의한 순환점 을 통과하기 때문에 그렇게 이름이 붙여졌다. 이 9가지 포인트는 다음과 같다.[31] [32]
삼각형 각 변의 중간점 각 고도 의 발삼각형의 각 꼭지점 에서 직교점 까지의 선 세그먼트 의 중간점(세 개의 고도가 만나는 곳, 이 선 세그먼트는 각각의 고도에 위치함) 1822년에 칼 푸에르바흐는 어떤 삼각형의 9점 원도 그 삼각형의 3개 원근에 외부적으로 접하고 내적으로 그 근방에 접한다는 것을 발견했다; 이 결과는 푸에르바흐의 정리 라고 알려져 있다. 그는 다음과 같은 사실을 증명했다.[citation needed
... 삼각형 고도의 발을 통과하는 원은 4개의 원 모두에 접하고, 그 원은 삼각형의 세 면에 접하고 있다... (Feuerbach 1822 ) harv 오류: 대상 없음: CITREFuerbach1822 (도움말 ) 근친과 9점 원과 접하는 삼각형 중심 을 푸에르바흐 포인트 라고 한다.
인센티브 및 중심 외 삼각형 세그먼트 B C {\displaystyle BC C A displaystyle CA} 및 A displaystyle AB} 인센티브 삼각형 의 정점이다. 인센티브 삼각형의 정점에 대한 3행 좌표는 다음과[citation needed 같다.
( 정반대 A ) = 0 : 1 : 1 {\displaystyle \left({\text{vertex 반대쪽}\,A\right)=0:1:1}} ( 정반대 B ) = 1 : 0 : 1 {\displaystyle \left({\text{vertex 반대쪽}\,B\right)=1:0:1} ( 정반대 C ) = 1 : 1 : 0. {\displaystyle \왼쪽({\text{vertex 반대쪽}\,C\오른쪽)=1:1:0. } 기준 삼각형의 중심 바깥쪽 삼각형은 기준 삼각형의 중심부에 정점이 있다. 옆면은 기준 삼각형의 외부 각도 이등분선에 있다(페이지 상단 그림 참조). 중심 바깥 삼각형의 정점에 대한 삼선 좌표는 다음과[citation needed 같다.
( 정반대 A ) = − 1 : 1 : 1 {\displaystyle({\text{vertex 반대쪽}\,A)=-1:1:1:1} ( 정반대 B ) = 1 : − 1 : 1 {\displaystyle({\text{vertex 반대쪽}\,B)=1:-1:1} ( 정반대 C ) = 1 : 1 : − 1. {\displaystyle({\text{vertex 반대쪽}\,C)=1:1:1:1-1.} 원 네 개에 대한 방정식 Let x : y : z {\displaystyle x:y:z} trilinear coordinates , and let u = cos 2 ( A / 2 ) {\displaystyle u=\cos ^{2}\left(A/2\right)} v = cos 2 ( B / 2 ) {\displaystyle v=\cos ^{2}\left(B/2\right)} w = cos 2 ( C / 2 ) {\displaystyle w=\cos ^{2}\left(C/2\right)} [33] : 210–215
근친: u 2 x 2 + v 2 y 2 + w 2 z 2 − 2 v w y z − 2 w u z x − 2 u v x y = 0 ± x cas ( A 2 ) ± y cas ( B 2 ) ± z cas ( C 2 ) = 0 {\displaystyle {\begin{aligned}u^{2}x^{2}+v^{2}y^{2}+w^{2}z^{2}-2vwyz-2wuzx-2uvxy&=0\\\pm {\sqrt {x}}\cos \left({\frac {A}{2}}\right)\pm {\sqrt {y}}\cos \left({\frac {B}{2}}\right)\pm {\sqrt {z}}\cos \left({\frac {C}{2}}\right)&=0\end{aligned}}} A {\displaystyle A} u 2 x 2 + v 2 y 2 + w 2 z 2 − 2 v w y z + 2 w u z x + 2 u v x y = 0 ± − x cas ( A 2 ) ± y cas ( B 2 ) ± z cas ( C 2 ) = 0 {\displaystyle {\begin{aligned}u^{2}x^{2}+v^{2}y^{2}+w^{2}z^{2}-2vwyz+2wuzx+2uvxy&=0\\\pm {\sqrt {-x}}\cos \left({\frac {A}{2}}\right)\pm {\sqrt {y}}\cos \left({\frac {B}{2}}\right)\pm {\sqrt {z}}\cos \left({\frac {C}{2}}\right)&=0\end{aligned}}} B {\displaystyle B} u 2 x 2 + v 2 y 2 + w 2 z 2 + 2 v w y z − 2 w u z x + 2 u v x y = 0 ± x cas ( A 2 ) ± − y cas ( B 2 ) ± z cas ( C 2 ) = 0 {\displaystyle {\begin{aligned}u^{2}x^{2}+v^{2}y^{2}+w^{2}z^{2}+2vwyz-2wuzx+2uvxy&=0\\\pm {\sqrt {x}}\cos \left({\frac {A}{2}}\right)\pm {\sqrt {-y}}\cos \left({\frac {B}{2}}\right)\pm {\sqrt {z}}\cos \left({\frac {C}{2}}\right)&=0\end{aligned}}} C {\displaystyle C} u 2 x 2 + v 2 y 2 + w 2 z 2 + 2 v w y z + 2 w u z x − 2 u v x y = 0 ± x cas ( A 2 ) ± y cas ( B 2 ) ± − z cas ( C 2 ) = 0 {\displaystyle {\begin{aligned}u^{2}x^{2}+v^{2}y^{2}+w^{2}z^{2}+2vwyz+2wuzx-2uvxy&=0\\\pm {\sqrt {x}}\cos \left({\frac {A}{2}}\right)\pm {\sqrt {y}}\cos \left({\frac {B}{2}}\right)\pm {\sqrt {-z}}\cos \left({\frac {C}{2}}\right)&=0\end{aligned}}} 오일러의 정리 오일러의 정리 에는 삼각형으로 다음과 같이 되어 있다.
( R − r ) 2 = d 2 + r 2 , {\displaystyle (R-r)^{2}=d^{2}+r^{2},} 여기 R {\displaystyle R} {\displaystyle r} displaystyle }
excircle의 경우 등식이 유사하다.
( R + r 이외의 ) 2 = d 이외의 2 + r 이외의 2 , {\displaystyle \left(R+r_{\text{ex}\오른쪽)^{2}=d_{\text{ex}^{2}+r_{\text}^{ex}}}} 여기서 r ex {\ displaystyle r_{\text{ex}} d ex {\ displaystyle d_{\text{ex}}} [34] [35] [36]
다른 다각형에 대한 일반화 일부(그러나 전부는 아님) 사변측정감시자들은 근친상간이다. 이것을 접선 사변측정감시 라고 한다. 그들의 많은 속성들 중에서 아마도 가장 중요한 것은 두 쌍의 반대편이 같은 합을 가지고 있다는 것이다. 이것을 피토 정리 라고 한다.[citation needed
보다 일반적으로, 각 면에 접하는 원(즉, 각 면에 접하는 원)이 새겨진 변의 수를 임의로 갖는 다각형을 접선 다각형 이라고 한다.[citation needed
참고 항목 메모들 ^ 케이(1969 , 페이지 140)^ 앨츠힐러 코트(1925 , 페이지 74)^ a b c d e 앨츠힐러 코트(1925 , 페이지 73)^ 케이 (1969년 , 페이지 117년)^ a b c 존슨, 로저 A, 어드밴스트 유클리드 기하학 , 도버, 2007년 (기원. 1929년) ^ a b Triangle Centers 백과사전 웨이백머신 에 보관 된 2012-04-19 2014-10-28^ 케이(1969 , 페이지 201)^ Allaire, Patricia R.; Zhou, Junmin; Yao, Haishen (March 2012), "Proving a nineteenth century ellipse identity", Mathematical Gazette 96 : 161–165 ^ Altshiller-Court, Nathan (1980), College Geometry , Dover Publications^ 2003년 7월 수학 가제트 , 323-324. ^ 추, 토마스, 펜타곤 , 2005년 봄, 페이지 45, 문제 584. ^ 케이(1969 , 페이지 203)^ a b c d 벨, 에이미, "한센의 오른쪽 삼각형 정리, 그 반대와 일반화", 포럼 기하학 6, 2006, 335–342. ^ 코도코스타스, 디미트리오스, "트라이앵글 이퀄라이저", 수학 매거진 83, 2010년 4월 페이지 141-146. ^ 앨리어, 패트리샤 R.; 저우, 준민; 그리고 야오, 하이젠, "19세기 타원 정체성 증명" 수학적 가제트 ^ 알츠힐러 코트, 네이쓴 대학 기하학 , 도버 출판물, 1980. ^ 포사멘티어, 알프레드 S, 레만, 잉그마르. 프로메테우스 북스, 2012년 삼각형 비밀 ^ a b c Franzsen, William N. (2011). "The distance from the incenter to the Euler line" (PDF) . Forum Geometricorum . 11 : 231–236. MR 2877263 . ^ 콕시터, H.S.M. "지오메트리 2차 에디션 소개. 1961년 와일리 ^ 민다, D, 펠프스, S, "삼각형, 타원형, 입방형 다항식", 미국 수학 2008년 정리 4.1. ^ 와이스슈타인, 에릭 W. "컨택트 트라이앵글" Wolfram Web Resource에서 온. http://mathworld.wolfram.com/ContactTriangle.html ^ 크리스토퍼 J. 브래들리와 제프 C. 스미스, "삼각형의 중심지" 포럼 기하학 http://forumgeom.fau.edu/FG2006volume6/FG200607index.html ^ Dekov, Deko (2009). "Computer-generated Mathematics : The Gergonne Point" (PDF) . Journal of Computer-generated Euclidean Geometry . 1 : 1–14. Archived from the original (PDF) on 2010-11-05. ^ 앨츠힐러 코트(1925 , 페이지 74)^ 앨츠힐러 코트(1925 , 페이지 79)^ 케이 (1969년 , 페이지 202^ 앨츠힐러 코트(1925 , 페이지 79)^ 베이커, 마커스, "평면 삼각형 면적에 대한 공식 모음," 수학 연혁 , 제1부 1885년 1월(6), 제1부 134-138. (제2부(1), 제2부 1885년 9월-18일 참조) ^ 그린버그, 다리즈, 유, 바울, "터커 서클로서의 아폴로니우스 서클", 포럼 기하학 2, 2002: 175-182 페이지. ^ Stevanovic, Milorad R, "아폴로니우스 원과 관련 삼각형 중심", 포럼 기하학 3, 2003, 187-195. ^ 알츠힐러 코트(1925 , 페이지 103–110)^ 케이(1969 , 페이지 18, 245)^ 휘트워스, 윌리엄 앨런 잊혀진 책들, 2012년(원점)의 2차원의 현대 해석 기하학적 좌표 및 기타 방법 . Diighton, Bell, Co., 1866년). http://www.forgottenbooks.com/search?q=Trilinear+coordinates&t=books ^ 넬슨, 로저 "언어 없는 증거를 통한 오일러의 삼각 불평등", 수학잡지 81(1), 2008년 2월 58-61. ^ Johnson, R. A. Modern Geometry , Houghton Mifflin, 보스턴, 1929년: 페이지 187. ^ 레브 에멜랴노프, 타티아나 에멜랴노바. "오일러의 공식과 폰셀렛의 다공성", 포럼 기하학 1, 2001: 페이지 137–140.
참조 Altshiller-Court, Nathan (1925), College Geometry: An Introduction to the Modern Geometry of the Triangle and the Circle (2nd ed.), New York: Barnes & Noble , LCCN 52013504 Kay, David C. (1969), College Geometry , New York: Holt, Rinehart and Winston , LCCN 69012075 Kimberling, Clark (1998). "Triangle Centers and Central Triangles". Congressus Numerantium (129): i–xxv, 1–295. Kiss, Sándor (2006). "The Orthic-of-Intouch and Intouch-of-Orthic Triangles". Forum Geometricorum (6): 171–177. 외부 링크 상호적인 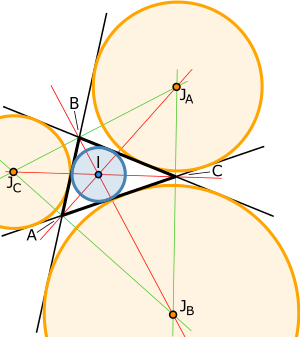






















 세 꼭지점의 각이다.
세 꼭지점의 각이다. 



























 옳다.
옳다. 






 ([
([

 .
. 








 원래 삼각형의 면적,
원래 삼각형의 면적, 














 되도록 하고, 그 중심은
되도록 하고, 그 중심은 
















 (가) 준다.
(가) 준다. 


 ,
, 

































 원곡선과 해당 excercle의 중심 사이의 거리다.
원곡선과 해당 excercle의 중심 사이의 거리다.

